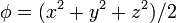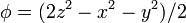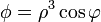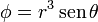Cálculo de laplacianos
De Laplace
(→Segundo campo) |
(→Tercer campo) |
||
| Línea 28: | Línea 28: | ||
===Tercer campo=== | ===Tercer campo=== | ||
| + | Para el tercer campo, ya tenemos su expresión en cilíndricas. Calculamos el laplaciano en estas coordenadas | ||
| + | |||
| + | <center><math>\nabla^2\phi = \frac{1}{\rho}\frac{\partial \ | ||
| + | }{\partial \rho}\left(\rho\frac{\partial \phi}{\partial \rho}\right)+ | ||
| + | \frac{1}{\rho^2}\frac{\partial^2 \phi}{\partial \varphi^2}+\frac{\partial^2 \phi}{\partial z^2} = | ||
| + | = 9\rho\cos\varphi - \rho\cos\varphi = 8\rho\cos\varphi</math></center> | ||
| + | |||
| + | En cartesianas este campo se expresa | ||
| + | |||
| + | <center><math>\phi = (x^2+y^2)x = x^3 + y^2x</math></center> | ||
| + | |||
| + | y su laplaciano vale | ||
| + | |||
| + | <center><math>\nabla^2\phi = 6x + 2x = 8x</math></center> | ||
| + | |||
| + | En esféricas, la expresión del campo es | ||
| + | |||
| + | <center><math>\phi = r^3\mathrm{sen}^3\theta\cos\varphi</math></center> | ||
| + | |||
| + | y la del laplaciano, separando previamente los sumandos, | ||
| + | |||
| + | <center><math>\frac{1}{r^2}\frac{\partial \ }{\partial r}\left(r^2\frac{\partial \phi}{\partial r}\right) = | ||
| + | 12r\,\mathrm{sen}^3\theta\cos\varphi</math></center> | ||
| + | |||
| + | |||
| + | <center><math>\frac{1}{r^2\mathrm{sen}\,\theta}\frac{\partial \ }{\partial \theta}\left(\mathrm{sen}\,\theta\frac{\partial \phi}{\partial \theta}\right) = | ||
| + | r\left(9\mathrm{sen}\,\theta\cos^2\theta-3\mathrm{sen}^3\theta\right)\cos\varphi | ||
| + | = r\left(9\mathrm{sen}\,\theta-12\mathrm{sen}^3\theta\right)\cos\varphi | ||
| + | </math></center> | ||
| + | |||
| + | <center><math>\frac{1}{r^2\mathrm{sen}^2\theta}\frac{\partial^2 \phi}{\partial \varphi^2} = -r\,\mathrm{sen}\,\theta\cos\varphi</math></center> | ||
| + | |||
| + | <center><math>\nabla^2\phi = 8r\,\mathrm{sen}\,\theta\cos\varphi</math></center> | ||
| + | |||
| + | Los tres resultados son naturalmente coincidentes. | ||
| + | |||
===Cuarto campo=== | ===Cuarto campo=== | ||
[[Categoría:Problemas de fundamentos matemáticos]] | [[Categoría:Problemas de fundamentos matemáticos]] | ||
Revisión de 09:33 25 sep 2008
Contenido |
1 Enunciado
Calcule el laplaciano de los campos escalares
empleando coordenadas cartesianas, cilíndricas y esféricas.
2 Solución
2.1 Primer campo
El laplaciano se define como la divergencia del gradiente. Para el campo (1) se ve en el problema de cálculo de gradientes que su gradiente vale
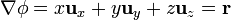
Hallar el laplaciano de 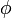 equivale a calcular la divergencia del vector de posición. Pero este cálculo ya se hace en el problema de cálculo de divergencias y rotacionales. Allí se ve que, independientemente del sistema empleado para calcularla
equivale a calcular la divergencia del vector de posición. Pero este cálculo ya se hace en el problema de cálculo de divergencias y rotacionales. Allí se ve que, independientemente del sistema empleado para calcularla
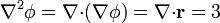
2.2 Segundo campo
Para el segundo campo, de nuevo calculamos su gradiente en un problema y la divergencia de éste en otro, donde se ve que

2.3 Tercer campo
Para el tercer campo, ya tenemos su expresión en cilíndricas. Calculamos el laplaciano en estas coordenadas
En cartesianas este campo se expresa
y su laplaciano vale

En esféricas, la expresión del campo es
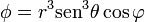
y la del laplaciano, separando previamente los sumandos,
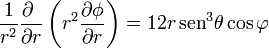
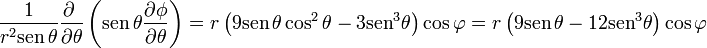
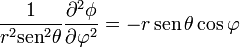

Los tres resultados son naturalmente coincidentes.