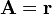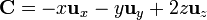Cálculo de divergencias y rotacionales

De Laplace

Contenido

1 Enunciado
2 Campo A
- 2.1 Divergencia
- 2.2 Rotacional
3 Campo B
- 3.1 Divergencia
- 3.2 Rotacional
4 Campo C
- 4.1 Divergencia
- 4.2 Rotacional
5 Campo D

1 Enunciado

Para los campos vectoriales

$\mathbf{A} = \mathbf{r}\,$
$\mathbf{B}=-y\mathbf{u}_{x}+x\mathbf{u}_{y}\,$
$\mathbf{C} = -x\mathbf{u}_{x}-y\mathbf{u}_{y}+2z\mathbf{u}_{z}\,$
$\mathbf{D} = \rho^2\cos\varphi\,\mathbf{u}_{\rho}+\rho^2\,\mathrm{sen}\,\varphi\,\mathbf{u}_{\varphi}$

calcule su divergencia y su rotacional, empleando en cada caso, coordenadas cartesianas, cilíndricas y esféricas. ¿Cuáles son irrotacionales y cuáles solenoidales?

2 Campo A

2.1 Divergencia

La divergencia, calculada en cartesianas, del vector de posición, es

$\nabla{\cdot}\mathbf{A} = \frac{\partial x}{\partial x}+\frac{\partial y}{\partial y}+\frac{\partial z}{\partial z} = 3$

Para este mismo campo, en cilíndricas, sustituyendo la expresión de 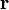$\mathbf{r}$ dada en otro problema

$\nabla{\cdot}\mathbf{A} = \frac{1}{\rho}\frac{\partial \rho^2}{\partial \rho}+\frac{\partial z}{\partial z} = \frac{2\rho}{\rho}+1 = 3$

y, en esféricas,

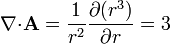
$\nabla{\cdot}\mathbf{A} = \frac{1}{r^2}\frac{\partial (r^3)}{\partial r}= 3$

2.2 Rotacional

Para el rotacional de este mismo campo, empleando coordenadas cartesianas

$\nabla\times\mathbf{A} = \left|\begin{matrix} \mathbf{u}_{x} & \mathbf{u}_{y} & \mathbf{u}_{z} \\ & & \\ \displaystyle\frac{\partial \ }{\partial x} & \displaystyle\frac{\partial \ }{\partial y} & \displaystyle\frac{\partial \ }{\partial z} \\ && \\ x & y & z \end{matrix}\right| = 0\mathbf{u}_{x}+0\mathbf{u}_{y}+0\mathbf{u}_{z}=\mathbf{0}$

en cilíndricas

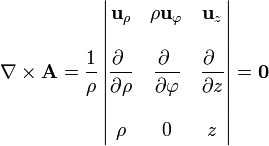
$\nabla\times\mathbf{A} = \frac{1}{\rho}\left|\begin{matrix}\mathbf{u}_{\rho} & \rho\mathbf{u}_{\varphi} & \mathbf{u}_{z} \\ & & \\ \displaystyle\frac{\partial \ }{\partial \rho} &\displaystyle\frac{\partial \ }{\partial \varphi} & \displaystyle\frac{\partial \ }{\partial z} \\ && \\ \rho & 0 & z\end{matrix}\right|= \mathbf{0}$

y en esféricas

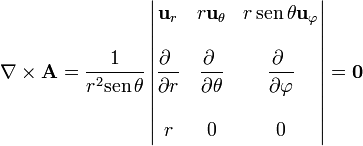
$\nabla\times\mathbf{A} = \frac{1}{r^2\mathrm{sen}\,\theta}\left|\begin{matrix}\mathbf{u}_{r} & r\mathbf{u}_{\theta} & r\,\mathrm{sen}\,\theta\mathbf{u}_{\varphi} \\ & & \\ \displaystyle \frac{\partial \ }{\partial r} &\displaystyle\frac{\partial \ }{\partial \theta} & \displaystyle\frac{\partial \ }{\partial \varphi} \\ && \\ r & 0 & 0\end{matrix}\right| = \mathbf{0}$

Naturalmente los resultados son los mismos independientemente del sistema empleado para calcularlos.

3 Campo B

3.1 Divergencia

Para el segundo campo, su divergencia, calculada en cartesianas,

$\nabla{\cdot}\mathbf{B} = \frac{\partial (-y)}{\partial x}+\frac{\partial x}{\partial y}+\frac{\partial 0}{\partial z}=0$

En cilíndricas este campo se escribe

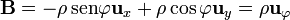
$\mathbf{B} = -\rho\,\mathrm{sen}\varphi\mathbf{u}_{x}+\rho\cos\varphi\mathbf{u}_{y}=\rho\mathbf{u}_{\varphi}$

y la divergencia

$\nabla{\cdot}\mathbf{B} = 0+ \frac{1}{\rho}\frac{\partial \rho}{\partial \varphi}+0 = 0$

En esféricas el campo es

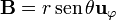
$\mathbf{B} = r \,\mathrm{sen}\,\theta\mathbf{u}_{\varphi}$

y la divergencia

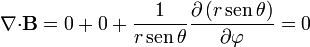
$\nabla{\cdot}\mathbf{B} = 0+0+\frac{1}{r\,\mathrm{sen}\,\theta}\frac{\partial \left(r\,\mathrm{sen}\,\theta\right)}{\partial \varphi} = 0$

3.2 Rotacional

Para el rotacional, en cartesianas,

$\nabla\times\mathbf{B} = \left|\begin{matrix} \mathbf{u}_{x} & \mathbf{u}_{y} & \mathbf{u}_{z} \\ & & \\ \displaystyle\frac{\partial \ }{\partial x} & \displaystyle\frac{\partial \ }{\partial y} & \displaystyle\frac{\partial \ }{\partial z} \\ && \\ -y & x & 0\end{matrix}\right|= 0\mathbf{u}_{x}+0\mathbf{u}_{y}+2\mathbf{u}_{z}=2\mathbf{u}_{z}$

En cilíndricas

$\nabla\times\mathbf{B} = \frac{1}{\rho}\left|\begin{matrix}\mathbf{u}_{\rho} & \rho\mathbf{u}_{\varphi} & \mathbf{u}_{z} \\ & & \\ \displaystyle\frac{\partial \ }{\partial \rho} &\displaystyle\frac{\partial \ }{\partial \varphi} & \displaystyle\frac{\partial \ }{\partial z} \\ && \\ 0 & \rho^2 & 0\end{matrix}\right|= 2\mathbf{u}_{z}$

y en esféricas

$\nabla\times\mathbf{B} = \frac{1}{r^2\mathrm{sen}\,\theta}\left|\begin{matrix}\mathbf{u}_{r} & r\mathbf{u}_{\theta} & r\,\mathrm{sen}\,\theta\mathbf{u}_{\varphi} \\ & & \\ \displaystyle \frac{\partial \ }{\partial r} &\displaystyle\frac{\partial \ }{\partial \theta} & \displaystyle\frac{\partial \ }{\partial \varphi} \\ && \\ 0 & 0 & r^2\mathrm{sen}^2\theta\end{matrix}\right|= 2\cos\theta\mathbf{u}_{r}-2\,\mathrm{sen}\,\theta\mathbf{u}_{\theta}$

De nuevo el resultado es el mismo aunque, al estar expresado en base diferentes, parece formalmente distinto.

4 Campo C

4.1 Divergencia

Para el tercer campo, la divergencia en cartesianas

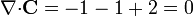
$\nabla{\cdot}\mathbf{C} = -1-1+2 = 0$

En cilíndricas, aplicando los resultados del problema de cálculo de gradientes

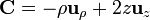
$\mathbf{C} = -\rho\mathbf{u}_{\rho}+2z\mathbf{u}_{z}$

y el cálculo de la divergencia da

$\nabla{\cdot}\mathbf{C} = -\frac{1}{\rho}\frac{\partial \left(\rho^2\right)}{\partial \rho}+\frac{\partial (2z)}{\partial z}= -2 + 2 = 0$

y en esféricas

$\mathbf{C} = r(3\cos^2\theta-1)\mathbf{u}_{r}-3r\,\mathrm{sen}\,\theta\cos\theta\mathbf{u}_{\theta}$

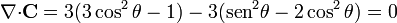
$\nabla{\cdot}\mathbf{C} = 3(3\cos^2\theta-1)-3(\mathrm{sen}^2\theta-2\cos^2\theta)=0$

4.2 Rotacional

Para el rotacional, en cartesianas

$\nabla\times\mathbf{C} = \left|\begin{matrix} \mathbf{u}_{x} & \mathbf{u}_{y} & \mathbf{u}_{z} \\ & & \\ \displaystyle\frac{\partial \ }{\partial x} & \displaystyle\frac{\partial \ }{\partial y} & \displaystyle\frac{\partial \ }{\partial z} \\ && \\ -x & -y & 2z\end{matrix}\right|= \mathbf{0}$

En cilíndricas

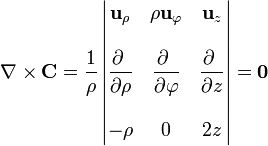
$\nabla\times\mathbf{C} = \frac{1}{\rho}\left|\begin{matrix}\mathbf{u}_{\rho} & \rho\mathbf{u}_{\varphi} & \mathbf{u}_{z} \\ & & \\ \displaystyle\frac{\partial \ }{\partial \rho} &\displaystyle\frac{\partial \ }{\partial \varphi} & \displaystyle\frac{\partial \ }{\partial z} \\ && \\ -\rho & 0 & 2z\end{matrix}\right|= \mathbf{0}$

y en esféricas

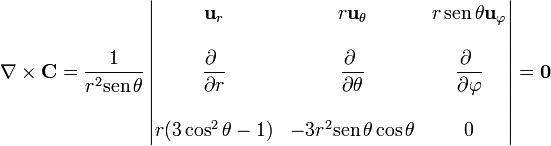
$\nabla\times\mathbf{C} = \frac{1}{r^2\mathrm{sen}\,\theta}\left|\begin{matrix}\mathbf{u}_{r} & r\mathbf{u}_{\theta} & r\,\mathrm{sen}\,\theta\mathbf{u}_{\varphi} \\ & & \\ \displaystyle \frac{\partial \ }{\partial r} &\displaystyle\frac{\partial \ }{\partial \theta} & \displaystyle\frac{\partial \ }{\partial \varphi} \\ && \\ r(3\cos^2\theta-1) & -3r^2\mathrm{sen}\,\theta\cos\theta & 0\end{matrix}\right|= \mathbf{0}$

5 Campo D

Por último, para el campo

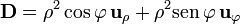
$\mathbf{D} = \rho^2\cos\varphi\,\mathbf{u}_{\rho}+\rho^2\mathrm{sen}\,\varphi\,\mathbf{u}_{\varphi}$

calculamos en primer lugar su divergencia y su rotacional en cilíndricas, ya que en estas coordenadas viene expresado el campo.

$\nabla{\cdot}\mathbf{D} = 3\rho\cos\varphi +\rho\cos\varphi = 4\rho\cos\varphi$ $\nabla\times\mathbf{D} = \frac{1}{\rho}\left|\begin{matrix}\mathbf{u}_{\rho} & \rho\mathbf{u}_{\varphi} & \mathbf{u}_{z} \\ & & \\ \displaystyle\frac{\partial \ }{\partial \rho} &\displaystyle\frac{\partial \ }{\partial \varphi} & \displaystyle\frac{\partial \ }{\partial z} \\ && \\ \rho^2\cos\varphi & \rho^3\mathrm{sen}\varphi & 0\end{matrix}\right| = 4\rho\mathrm{sen}\varphi\mathbf{u}_{z}$

Para calcular estas cantidades en cartesianas, pasamos el campo a este sistema

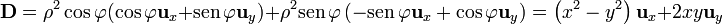
$\mathbf{D} = \rho^2\cos\varphi(\cos\varphi\mathbf{u}_{x}+\mathrm{sen}\,\varphi\mathbf{u}_{y})+\rho^2\mathrm{sen}\,\varphi\left(-\mathrm{sen}\,\varphi\mathbf{u}_{x}+\cos\varphi\mathbf{u}_{y}\right) =\left(x^2-y^2\right)\mathbf{u}_{x}+2xy\mathbf{u}_{y}$

y calculamos su divergencia

$\nabla{\cdot}\mathbf{D} = 2x+2x = 4x$

y su rotacional

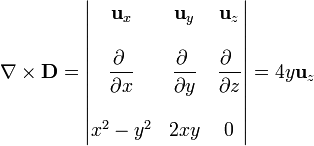
$\nabla\times\mathbf{D} = \left|\begin{matrix} \mathbf{u}_{x} & \mathbf{u}_{y} & \mathbf{u}_{z} \\ & & \\ \displaystyle\frac{\partial \ }{\partial x} & \displaystyle\frac{\partial \ }{\partial y} & \displaystyle\frac{\partial \ }{\partial z} \\ && \\ x^2-y^2 & 2xy & 0\end{matrix}\right|= 4y\mathbf{u}_{z}$

Para pasar a esféricas, primero expresamos 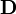$\mathbf{D}$ en sus componentes cartesianas

$\mathbf{D}=(x^2-y^2)\mathbf{u}_{x}+2xy\mathbf{u}_{y} =r^2\mathrm{sen}^2\theta\cos(2\varphi)\mathbf{u}_{x}+ r^2\mathrm{sen}^2\theta\,\mathrm{sen}(2\varphi)\mathbf{u}_{y}$

A continuación hallamos las diferentes componentes en esféricas

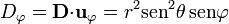
$D_r = \mathbf{D}{\cdot}\mathbf{u}_{r}=r^2\mathrm{sen}^3\theta\cos\varphi$ $D_\theta = \mathbf{D}{\cdot}\mathbf{u}_{\theta}=r^2\mathrm{sen}^2\theta\cos\theta\cos\varphi$ $D_\varphi = \mathbf{D}{\cdot}\mathbf{u}_{\varphi}=r^2\mathrm{sen}^2\theta\,\mathrm{sen}\varphi$

Luego, calculamos los diferentes sumandos que constituyen la divergencia

$\frac{1}{r^2}\frac{\partial \ }{\partial r}\left(r^2 D_r\right)=4r\,\mathrm{sen}^3\theta\cos\varphi$

$\frac{1}{r\,\mathrm{sen}\,\theta}\frac{\partial \ }{\partial \theta}\left(\mathrm{sen}\theta D_\theta\right)=r\cos\varphi(3\,\mathrm{sen}\theta\cos^2\theta-\mathrm{sen}^3\theta)$

$\frac{1}{r\,\mathrm{sen}\,\theta}\frac{\partial \ }{\partial \varphi}(D_\varphi)=r\,\mathrm{sen}\,\theta\cos\varphi$

y, por último, sumamos

$\nabla{\cdot}\mathbf{D}=4r\,\mathrm{sen}\,\theta\cos\varphi$

Para el rotacional

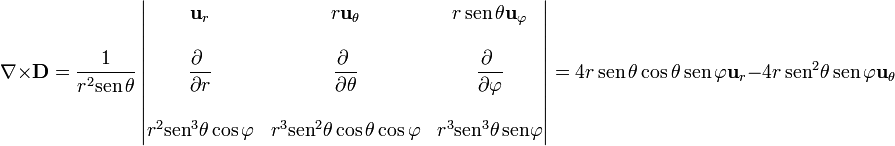
$\nabla\times\mathbf{D} = \frac{1}{r^2\mathrm{sen}\,\theta}\left|\begin{matrix}\mathbf{u}_{r} & r\mathbf{u}_{\theta} & r\,\mathrm{sen}\,\theta\mathbf{u}_{\varphi} \\ & & \\ \displaystyle \frac{\partial \ }{\partial r} &\displaystyle\frac{\partial \ }{\partial \theta} & \displaystyle\frac{\partial \ }{\partial \varphi} \\ && \\ r^2\mathrm{sen}^3\theta\cos\varphi & r^3\mathrm{sen}^2\theta\cos\theta\cos\varphi & r^3\mathrm{sen}^3\theta\,\mathrm{sen}\varphi\end{matrix}\right|= 4r\,\mathrm{sen}\,\theta\cos\theta\,\mathrm{sen}\,\varphi\mathbf{u}_{r}-4r\,\mathrm{sen}^2\theta\,\mathrm{sen}\,\varphi\mathbf{u}_{\theta}$

Teniendo en cuenta que

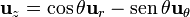
$y=\rho\,\mathrm{sen}\,\varphi=r\,\mathrm{sen}\theta\,\mathrm{sen}\varphi$ $\mathbf{u}_{z}=\cos\theta\mathbf{u}_{r}-\mathrm{sen}\,\theta\mathbf{u}_{\theta}$

puede verse que los tres resultados son coincidentes.

Cálculo de divergencias y rotacionales

De Laplace

Contenido

1 Enunciado

2 Campo A

2.1 Divergencia

2.2 Rotacional

3 Campo B

3.1 Divergencia

3.2 Rotacional

4 Campo C

4.1 Divergencia

4.2 Rotacional

5 Campo D

Herramientas:

Buscar

Vistas

Herramientas

Herramientas personales

Navegación

SEARCH

TOOLBOX

LANGUAGES