Coordenadas esféricas. Diferenciales
De Laplace
(Diferencias entre revisiones)
(→Enlaces) |
(→Diferenciales de superficie) |
||
| Línea 5: | Línea 5: | ||
==Diferenciales de superficie== | ==Diferenciales de superficie== | ||
| + | Dependiendo de la [[Coordenadas esféricas. Líneas y superficies coordenadas|coordenada que consideremos constante]], tenemos tres vectores diferenciales de superficie: | ||
| + | |||
| + | *Superficie <math>r = \mathrm{cte}</math> (superficies esféricas) | ||
| + | |||
| + | <center><math>\mathrm{d}\mathbf{S}_r = r^2\mathrm{sen}\,\theta\,\mathrm{d}\theta\,\mathrm{d}\varphi\,\mathbf{u}_r</math></center> | ||
| + | |||
| + | *Superficie <math>\theta = \mathrm{cte}</math> (conos) | ||
| + | |||
| + | <center><math>\mathrm{d}\mathbf{S}_\theta = r\,\mathrm{d}r\,\mathrm{d}\varphi\,\mathbf{u}_\theta</math></center> | ||
| + | |||
| + | *Superficie <math>\varphi = \mathrm{cte}</math> (semiplanos verticales) | ||
| + | |||
| + | <center><math>\mathrm{d}\mathbf{S}_\varphi = r\,\mathrm{d}r\,\mathrm{d}\theta\,\mathbf{u}_\varphi</math></center> | ||
==Diferencial de volumen== | ==Diferencial de volumen== | ||
Revisión de 19:58 22 nov 2007
Contenido |
1 Diferencial de camino
Aplicando la expresión general del diferencial de camino resulta
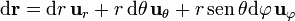
2 Diferenciales de superficie
Dependiendo de la coordenada que consideremos constante, tenemos tres vectores diferenciales de superficie:
- Superficie r = cte (superficies esféricas)
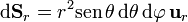
- Superficie θ = cte (conos)
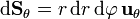
- Superficie
 (semiplanos verticales)
(semiplanos verticales)
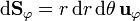
3 Diferencial de volumen
4 Enlaces
- Siguiente: : Como se hace una integral
- Anterior: Coordenadas cilíndricas. Diferenciales





