Coordenadas esféricas. Diferenciales
De Laplace
Contenido |
1 Diferencial de camino
Aplicando la expresión general del diferencial de camino resulta
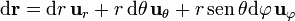
2 Diferenciales de superficie
Dependiendo de la coordenada que consideremos constante, tenemos tres vectores diferenciales de superficie:
- Superficie r = cte (superficies esféricas)
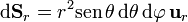
- Superficie θ = cte (conos)
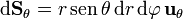
- Superficie
 (semiplanos verticales)
(semiplanos verticales)
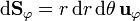
3 Diferencial de volumen
Combinando los tres diferenciales
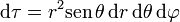
4 Enlaces
- Siguiente: Cómo se hace una integral
- Anterior: Coordenadas cilíndricas. Diferenciales





