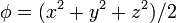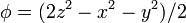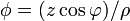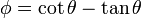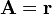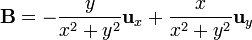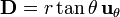Problemas de fundamentos matemáticos

Revisión a fecha de 08:31 23 sep 2008; Antonio (Discusión | contribuciones)

1 Campos escalares en diferentes sistemas

Exprese los siguientes campos escalares en coordenadas cartesianas, cilíndricas y esféricas

Exprese los siguientes campos vectoriales en coordenadas cartesianas, cilíndricas y esféricas:

$\mathbf{A} = \mathbf{r}\,$
$\mathbf{B} = -\dfrac{y}{x^2+y^2}\mathbf{u}_{x}+\dfrac{x}{x^2+y^2}\mathbf{u}_{y}$
$\mathbf{C} = 2\rho z\mathbf{u}_{\rho}-(\rho^2-z^2)\mathbf{u}_{z}$
$\mathbf{D}=r\tan\theta\,\mathbf{u}_{\theta}$