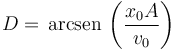Partícula con aceleración dependiente de x
De Laplace
(→Enunciado) |
|||
| Línea 27: | Línea 27: | ||
v\,\mathrm{d}v = a(x)\,\mathrm{d}x | v\,\mathrm{d}v = a(x)\,\mathrm{d}x | ||
\Longrightarrow | \Longrightarrow | ||
| - | \int v\,\mathrm{d}v = \int - | + | \int v\,\mathrm{d}v = \int -A^2x\,\mathrm{d}x |
\Longrightarrow | \Longrightarrow | ||
| - | \dfrac{1}{2}v^2 = -\dfrac{1}{2} | + | \dfrac{1}{2}v^2 = -\dfrac{1}{2}A^2x^2 + C |
</math> | </math> | ||
</center> | </center> | ||
| Línea 41: | Línea 41: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | v(x) = \sqrt{v_0^2 - | + | v(x) = \sqrt{v_0^2 - A^2x^2} |
</math> | </math> | ||
</center> | </center> | ||
| Línea 51: | Línea 51: | ||
\dfrac{\mathrm{d}x}{v(x)} = \mathrm{d}t | \dfrac{\mathrm{d}x}{v(x)} = \mathrm{d}t | ||
\Longrightarrow | \Longrightarrow | ||
| - | \dfrac{\mathrm{d}x}{\sqrt{v_0^2- | + | \dfrac{\mathrm{d}x}{\sqrt{v_0^2-A^2x^2}} = \mathrm{d}t |
</math> | </math> | ||
</center> | </center> | ||
| Línea 57: | Línea 57: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | x =\dfrac{v_0} | + | x =\dfrac{v_0}{A}\,\mathrm{sen}\,u |
\Longrightarrow | \Longrightarrow | ||
| - | \mathrm{d}x =\dfrac{v_0} | + | \mathrm{d}x =\dfrac{v_0}{A}\cos u\,\mathrm{d}u |
\Longrightarrow | \Longrightarrow | ||
| - | \dfrac{\mathrm{d}x}{\sqrt{v_0^2- | + | \dfrac{\mathrm{d}x}{\sqrt{v_0^2-A^2x^2}} |
= | = | ||
| - | \dfrac{\mathrm{d}u} | + | \dfrac{\mathrm{d}u}{A} |
</math> | </math> | ||
</center> | </center> | ||
| Línea 69: | Línea 69: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \int\dfrac{\mathrm{d}u} | + | \int\dfrac{\mathrm{d}u}{A} = \int \mathrm{d}t |
\Longrightarrow | \Longrightarrow | ||
| - | u = | + | u = At + D |
</math> | </math> | ||
</center> | </center> | ||
| Línea 77: | Línea 77: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | x = \dfrac{v_0} | + | x = \dfrac{v_0}{A}\,\mathrm{sen}\,(At + D) |
</math> | </math> | ||
</center> | </center> | ||
| Línea 83: | Línea 83: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | D = \,\mathrm{arcsen}\,\left(\dfrac{ | + | D = \,\mathrm{arcsen}\,\left(\dfrac{x_0A}{v_0}\right) |
</math> | </math> | ||
</center> | </center> | ||
[[Categoría:Problemas de cinemática del movimiento rectilíneo]] | [[Categoría:Problemas de cinemática del movimiento rectilíneo]] | ||
última version al 10:25 26 sep 2018
1 Enunciado
Una partícula se desplaza sobre el eje OX de modo que su aceleración cumple en cada instante a(x) = − A2x, siendo A una constante. En la posición inicial la velocidad de la partícula es v0. Determina la función v(x).
2 Solución
La aceleración es
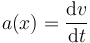
Introducimos la regla de la cadena multiplicando y dividiendo por dx
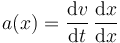
Como una derivada se puede entender como un cociente intercambiamos los dos números que aparecen en el denominador.
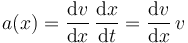
Hemos usado que v = dx / dt. Con esto nos ha quedado una ecuación diferencial en variables separables que se pueden integrar.
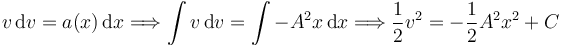
Imponiendo la condición inicial

y por tanto
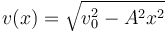
Ahora podemos plantear la ecuación diferencial para x(t)
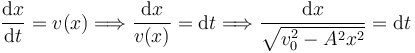
Para integrar hacemos el cambio
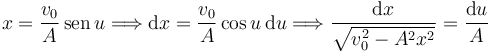
Integrando queda
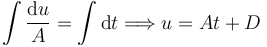
Y entonces
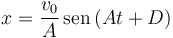
Si en t = 0 tenemos x = x0 nos queda