4.8. Rodadura permanente de un disco
De Laplace
(Diferencias entre revisiones)
(→Velocidades) |
(→Velocidades) |
||
| Línea 33: | Línea 33: | ||
<center><math>\overrightarrow{OB}=-R\vec{\imath}</math>{{qquad}}{{qquad}}<math>\vec{v}^B = \vec{v}^O +\vec{\omega}\times\overrightarrow{OB}=v_0\vec{\imath}+\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ 0 & 0 & -v_0/R \\ -R & 0 & 0 \end{matrix}\right| = v_0(\vec{\imath}+\vec{\jmath})</math></center> | <center><math>\overrightarrow{OB}=-R\vec{\imath}</math>{{qquad}}{{qquad}}<math>\vec{v}^B = \vec{v}^O +\vec{\omega}\times\overrightarrow{OB}=v_0\vec{\imath}+\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ 0 & 0 & -v_0/R \\ -R & 0 & 0 \end{matrix}\right| = v_0(\vec{\imath}+\vec{\jmath})</math></center> | ||
| + | |||
| + | Obtenemos que el punto de contacto con el suelo posee velocidad instantánea nula, mientras que el punto superior posee velocidad doble a la de avance de la rueda. | ||
| + | |||
| + | Las velocidades de los puntos O, B, C y D son perpendiculares al vector de posición relativo al punto A, como corresponde a que este se encuentre en el eje instantáneo de rotación: | ||
| + | |||
| + | <center><math>\vec{v}^P = \overbrace{\vec{v}^A}^{=\vec{0}} + \vec{\omega}\times\overrightarrow{AP}=\vec{\omega}\times\overrightarrow{AP}\perp\overrightarrow{AP}</math></center> | ||
==Aceleraciones== | ==Aceleraciones== | ||
[[Categoría:Problemas de cinemática del sólido rígido (G.I.T.I.)]] | [[Categoría:Problemas de cinemática del sólido rígido (G.I.T.I.)]] | ||
Revisión de 21:32 13 nov 2010
1 Enunciado
La rodadura permanente de un disco de radio R sobre una superficie horizontal puede describirse mediante el campo de velocidades

donde la superficie horizontal se encuentra en y = − R.
- Determine, para un instante dado, la velocidades de los puntos A, B, C y D situados en los cuatro cuadrantes del disco.
- Calcule la aceleración de estos puntos para el mismo instante, suponiendo v0 = cte.
2 Velocidades
Para cada uno de los puntos, basta aplicar la fórmula correspondiente
- Punto A
- Su vector de posición relativa es
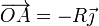
- por lo que su velocidad vale
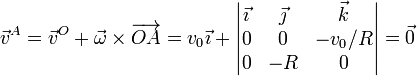
- Punto B
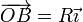
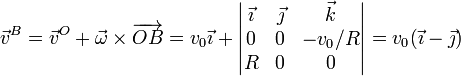
- Punto C

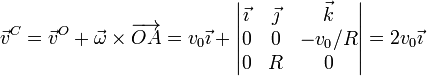
- Punto D
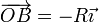
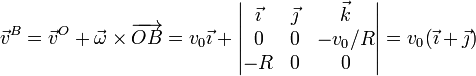
Obtenemos que el punto de contacto con el suelo posee velocidad instantánea nula, mientras que el punto superior posee velocidad doble a la de avance de la rueda.
Las velocidades de los puntos O, B, C y D son perpendiculares al vector de posición relativo al punto A, como corresponde a que este se encuentre en el eje instantáneo de rotación:







