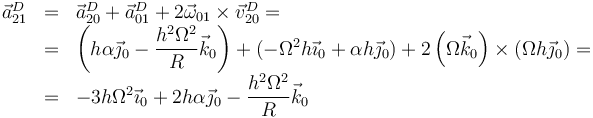Disco en varilla horizontal (CMR)
De Laplace
| (11 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
==Enunciado== | ==Enunciado== | ||
| - | Un disco de radio R (“sólido 2”) se encuentra ensartado mediante un rodamiento sin fricción en un eje horizontal de longitud h (“sólido 0”). Este eje está montado sobre un soporte vertical fijo de altura R. El disco rueda sin deslizar sobre la superficie horizontal <math>z=0</math> (“sólido 1”). Consideramos tres sistemas de referencia. Uno fijo en el suelo, uno ligado al disco, y uno intermedio en el que el eje <math>OX_0</math> es a lo largo de la barra horizontal y <math>OZ_0=OZ_1</math> en todo momento. Sea <math> | + | Un disco de radio R (“sólido 2”) se encuentra ensartado mediante un rodamiento sin fricción en un eje horizontal de longitud h (“sólido 0”). Este eje está montado sobre un soporte vertical fijo de altura <math>R</math>. El disco rueda sin deslizar sobre la superficie horizontal <math>z=0</math> (“sólido 1”). Consideramos tres sistemas de referencia. Uno fijo en el suelo, uno ligado al disco, y uno intermedio en el que el eje <math>OX_0</math> es a lo largo de la barra horizontal y <math>OZ_0=OZ_1</math> en todo momento. Sea <math>\theta(t)</math> el ángulo que el eje <math>OX_0</math> forma con el <math>OX_1</math>. En un instante dado <math>\theta=0\,</math>, <math>\dot{\theta}=\Omega</math>, <math>\ddot{\theta}=\alpha</math>. |
| - | <center>[[Archivo:disco-varilla-horizontal.png|600px]]</center> | + | <center>[[Archivo:disco-varilla-horizontal-02.png|600px]]</center> |
Para ese instante: | Para ese instante: | ||
# Determine los vectores <math>\vec{\omega}_{01}</math>, <math>\vec{\omega}_{20}</math> y <math>\vec{\omega}_{21}</math>. | # Determine los vectores <math>\vec{\omega}_{01}</math>, <math>\vec{\omega}_{20}</math> y <math>\vec{\omega}_{21}</math>. | ||
# Halle la posición de los ejes instantáneos de rotación en los movimientos {01}, {20} y {21}. | # Halle la posición de los ejes instantáneos de rotación en los movimientos {01}, {20} y {21}. | ||
| - | # Calcule las velocidades en el movimiento {21} y el {20} del punto | + | # Calcule las velocidades en el movimiento {21} y el {20} del punto A de contacto del disco con el suelo; del G, centro del disco, y de D, el punto más alto del disco. |
# Halle las aceleraciones angulares <math>\vec{\alpha}_{01}</math>, <math>\vec{\alpha}_{20}</math> y <math>\vec{\alpha}_{21}</math>. | # Halle las aceleraciones angulares <math>\vec{\alpha}_{01}</math>, <math>\vec{\alpha}_{20}</math> y <math>\vec{\alpha}_{21}</math>. | ||
| - | # Calcule las aceleraciones en los movimientos {21} y {20} de los puntos | + | # Calcule las aceleraciones en los movimientos {21} y {20} de los puntos A, G y D del apartado (3). |
==Velocidades angulares== | ==Velocidades angulares== | ||
| - | En este problema tenemos tres sistemas de | + | En este problema tenemos tres sistemas de referencia, los dos sólidos y el sistema intermedio. |
| - | + | Lo primero que observamos es que el punto O es fijo en los tres movimientos: En el {01} porque pertenece al eje de rotación <math>OZ_1</math>. En el {20} porque pertenece al eje <math>OX_0</math>, de rotación en este movimiento, y en el {21} por composición de velocidades | |
| - | El sistema 0 | + | <center><math>\vec{v}^O_{21}=\vec{v}^O_{20}+\vec{v}^O_{01}=\vec{0}+\vec{0}=\vec{0}</math></center> |
| + | |||
| + | Puesto que el punto O es fijo en los tres, podemos emplearlo como origen de coordenadas común. | ||
| + | |||
| + | El sistema “1” es el fijo, con ejes <math>OX_1</math> y <math>OY_1</math> horizontales y <math>OZ_1</math> vertical. | ||
| + | |||
| + | El sistema “0” tiene su eje <math>OX_0</math> siempre alineado con la varilla, siendo <math>OZ_0</math> vertical y coincidente con <math>OZ_1</math>. En el movimiento {01} este sistema gira en torno a <math>OZ_1=OZ_0</math> por lo que las bases respectivas cumplen | ||
<center><math>\begin{array}{rcl}\vec{\imath}_0&=&\cos(\theta)\vec{\imath}_1+\mathrm{sen}(\theta)\vec{\jmath}_1\\ | <center><math>\begin{array}{rcl}\vec{\imath}_0&=&\cos(\theta)\vec{\imath}_1+\mathrm{sen}(\theta)\vec{\jmath}_1\\ | ||
| Línea 25: | Línea 31: | ||
<center><math>\vec{\omega}_{01}=\dot{\theta}\vec{k}_1=\dot{\theta}\vec{k}_0</math></center> | <center><math>\vec{\omega}_{01}=\dot{\theta}\vec{k}_1=\dot{\theta}\vec{k}_0</math></center> | ||
| - | El sistema 2 es uno ligado al disco y cuyo eje <math>OX_2</math> coincide con el <math> | + | El sistema 2 es uno ligado al disco y cuyo eje <math>OX_2</math> coincide con el <math>OX_0</math>. El disco gira alrededor de este eje. Por tanto, la relación entre las correspondientes bases es |
<center><math>\begin{array}{rcl}\vec{\imath}_2&=&\vec{\imath}_0\\ | <center><math>\begin{array}{rcl}\vec{\imath}_2&=&\vec{\imath}_0\\ | ||
| Línea 42: | Línea 48: | ||
<center><math>\vec{\omega}_{21}=\vec{\omega}_{20}+\vec{\omega}_{01}=\dot{\psi}\vec{\imath}_0+\dot{\theta}\vec{k}_0</math></center> | <center><math>\vec{\omega}_{21}=\vec{\omega}_{20}+\vec{\omega}_{01}=\dot{\psi}\vec{\imath}_0+\dot{\theta}\vec{k}_0</math></center> | ||
| - | Obtenemos la relación entre las dos componentes imponiendo la condición de que la velocidad en el punto de contacto | + | Obtenemos la relación entre las dos componentes imponiendo la condición de que la velocidad en el punto A, de contacto entre el disco y el plano, es nula en el movimiento {21} |
| - | <center><math>\vec{0}=\vec{v}^ | + | <center><math>\vec{0}=\vec{v}^A_{21}=\overbrace{\vec{v}^O_{21}}^{=\vec{0}}+\vec{\omega}_{21}\times \overrightarrow{OA}</math></center> |
Desarrollando el producto vectorial | Desarrollando el producto vectorial | ||
| Línea 57: | Línea 63: | ||
<center><math>\vec{\omega}_{01}=\Omega\vec{k}_0\qquad\qquad \vec{\omega}_{20}=-\frac{h}{R}\Omega\vec{\imath}_0\qquad\qquad\vec{\omega}_{21}=\frac{\Omega\left(-h\vec{\imath}_0+R\vec{k}_0\right)}{R}</math></center> | <center><math>\vec{\omega}_{01}=\Omega\vec{k}_0\qquad\qquad \vec{\omega}_{20}=-\frac{h}{R}\Omega\vec{\imath}_0\qquad\qquad\vec{\omega}_{21}=\frac{\Omega\left(-h\vec{\imath}_0+R\vec{k}_0\right)}{R}</math></center> | ||
| + | |||
| + | ==Ejes instantáneos de rotación== | ||
| + | Lo localización de los tres EIR es sencilla: | ||
| + | ;Movimiento {01}: El eje es el <math>OZ_1=OZ_0</math> | ||
| + | ;Movimiento {20}: El eje es el <math>OX_2=OX_0</math> | ||
| + | ;Movimiento {21}: Es el que pasa por O y lleva la dirección de <math>\vec{\omega}_{21}</math> o, lo que es equivalente, el que pasa por O y A, al ser ambos puntos de velocidad nula en el movimiento {01}. | ||
| + | |||
| + | ==Velocidades lineales== | ||
| + | ===Del punto A=== | ||
| + | En el movimiento {21}, la velocidad del punto A es nula según hemos dicho. | ||
| + | |||
| + | <center><math>\vec{v}^A_{21}=\vec{0}</math></center> | ||
| + | |||
| + | En el movimiento {20} el punto A describe un movimiento circular en torno al eje del disco, que pasa por el origen O. | ||
| + | |||
| + | <center><math>\vec{v}^A_{20}=\vec{\omega}_{20}\times\overrightarrow{OA}=\left(-\frac{h}{R}\Omega\vec{\imath}_0\right)\times(h\vec{\imath}_0-R\vec{k}_0)=-\Omega h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | También se puede hallar esta velocidad a partir de la del movimiento {01} ya que por ser nula la del {21} | ||
| + | |||
| + | <center><math>\vec{v}^A_{20}=\vec{v}^A_{21}+\vec{v}^A_{10}=\vec{0}-\vec{v}^A_{01}=-\left(\Omega\vec{k}_0\right)\times(h\vec{\imath}_0-R\vec{k}_0)=-\Omega h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | ===Del punto G=== | ||
| + | En el movimiento {20} G está en reposo por pertenecer al eje de rotación | ||
| + | |||
| + | <center><math>\vec{v}^G_{20}=\vec{0}</math></center> | ||
| + | |||
| + | En el {21} y en el {01} realiza un movimiento de rotación alrededor de un eje que pasa por A. | ||
| + | |||
| + | <center><math>\vec{v}^G_{01}=\vec{\omega}_{01}\times\overrightarrow{OG}=(\Omega \vec{k}_0)\times (h\vec{\imath}_0)=-\Omega h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | Vemos que en este movimiento A y G tienen la misma velocidad por estar a la misma distancia del eje. De aquí | ||
| + | |||
| + | <center><math>\vec{v}^G_{21}=\vec{v}^G_{01}=-\Omega h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | ===Del punto D=== | ||
| + | Operando igualmente, tenemos en el movimiento {01} | ||
| + | |||
| + | <center><math>\vec{v}^D_{01}=\Omega h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | En el {20} | ||
| + | |||
| + | <center><math>\vec{v}^D_{20}=\left(-\frac{h}{R}\Omega\vec{\imath}_0\right)\times (h\vec{\imath}_0+R\vec{k}_0)=\Omega h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | y por tanto | ||
| + | |||
| + | <center><math>\vec{v}^D_{21}=\vec{v}^D_{21}+\vec{v}^D_{21}=2\Omega h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | ==Aceleraciones angulares== | ||
| + | El movimiento {01} es una rotación con eje fijo, siendo su aceleración angular | ||
| + | |||
| + | <center><math>\vec{\alpha}_{01}=\ddot{\theta}\vec{k}_0=\alpha\vec{k}_0</math></center> | ||
| + | |||
| + | Análogamente ocurre para el {20} | ||
| + | |||
| + | <center><math>\vec{\alpha}_{20}=\ddot{\psi}\vec{\imath}_0=-\frac{h}{R}\alpha\vec{\imath}_0</math></center> | ||
| + | |||
| + | Para el {21} empleamos la composición de aceleraciones angulares | ||
| + | |||
| + | <center><math>\vec{\alpha}_{21}=\vec{\alpha}_{20}+\vec{\alpha}_{01}+\vec{\omega}_{01}\times\vec{\omega}_{20}=-\frac{h}{R}\alpha\vec{\imath}_0+\alpha\vec{k}_0+\left(\Omega\vec{k}_0\right)\times\left(-\frac{h}{R}\Omega\vec{\imath}_0\right)=-\frac{h}{R}\alpha\vec{\imath}_0+\frac{h}{R}\Omega^2\vec{\jmath}_0+\alpha\vec{k}_0</math></center> | ||
| + | |||
| + | ==Aceleraciones lineales== | ||
| + | ===En el movimiento {01}=== | ||
| + | En el movimiento {01} todos los puntos describen un moviento circular acelerado alrededor del eje OZ<sub>1</sub>. Puesto que los tres puntos A, G y D, se hallan a la misma distancia del eje, la aceleración en este movimiento es igual para todos ellos | ||
| + | |||
| + | <center><math>\vec{a}^A_{01}=\vec{a}^G_{01}=\vec{a}^D_{01}=\vec{\alpha}_{01}\times\overrightarrow{OG}+\vec{\omega}_{01}\times(\vec{\omega}_{01}\times\overrightarrow{OG})</math></center> | ||
| + | |||
| + | Puesto que este movimiento es plano, podemos simplificar la expresión | ||
| + | |||
| + | <center><math>\vec{a}^G_{01}=\alpha\vec{k}_0\times(h\vec{\imath}_0)-\Omega^2(h\vec{\imath}_0)=-\Omega^2h\vec{\imath}_0+\alpha h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | ===En el movimiento {20}=== | ||
| + | Este movimiento es de rotación en torno al eje OX<sub>0</sub>, el cual pasa por G. Por tanto | ||
| + | |||
| + | <center><math>\vec{a}^G_{20}=\vec{0}</math></center> | ||
| + | |||
| + | Para el punto A, situado en el mismo plano director que G | ||
| + | |||
| + | <center><math>\vec{a}^A_{20}=\vec{a}^G_{20}+\vec{\alpha}_{20}\times\overrightarrow{GA}+\vec{\omega}_{20}\times(\vec{\omega}_{20}\times\overrightarrow{GA})</math></center> | ||
| + | |||
| + | lo que nos da | ||
| + | |||
| + | <center><math>\vec{a}^A_{20}=\left(-\frac{h\alpha}{R}\vec{\imath}_0\right)\times(-R\vec{k}_0)-\left(\frac{h\Omega}{R}\right)^2(-R\vec{k}_0)=-h\alpha\vec{\jmath}_0+\frac{h^2\Omega^2}{R}\vec{k}_0</math></center> | ||
| + | |||
| + | Para el punto D el cálculo es similar, invirtiendo el vector de posición relativa | ||
| + | |||
| + | <center><math>\vec{a}^A_{20}=\left(-\frac{h\alpha}{R}\vec{\imath}_0\right)\times(+R\vec{k}_0)-\left(\frac{h\Omega}{R}\right)^2(+R\vec{k}_0)=h\alpha\vec{\jmath}_0-\frac{h^2\Omega^2}{R}\vec{k}_0</math></center> | ||
| + | |||
| + | ===En el movimiento {21}=== | ||
| + | Aquí podemos calcularlas directamente con la fórmula análoga | ||
| + | |||
| + | <center><math>\vec{a}^P_{21}=\vec{a}^O_{21}+\vec{\alpha}_{21}\times\overrightarrow{OP}+\vec{\omega}_{21}\times(\vec{\omega}_{21}\times\overrightarrow{OP})</math></center> | ||
| + | |||
| + | o bien con el teorema de Coriolis | ||
| + | |||
| + | <center><math>\vec{a}^P_{21}=\vec{a}^P_{20}+\vec{a}^P_{01}+2\vec{\omega}_{01}\times\vec{v}^P_{20}</math></center> | ||
| + | |||
| + | Si empleamos el teorema de Coriolis, para el punto G resulta | ||
| + | |||
| + | <center><math>\vec{a}^G_{21}=\overbrace{\vec{a}^G_{20}}^{=\vec{0}}+\vec{a}^G_{01}+2\vec{\omega}_{01}\times\overbrace{\vec{v}^G_{20}}^{=\vec{0}}=\vec{a}^G_{01}=-\Omega^2h\vec{\imath}_0+\alpha h\vec{\jmath}_0</math></center> | ||
| + | |||
| + | Para el punto A | ||
| + | |||
| + | <center><math>\begin{array}{rcl}\vec{a}^A_{21}&=&\vec{a}^A_{20}+\vec{a}^A_{01}+2\vec{\omega}_{01}\times\vec{v}^A_{20}=\\ | ||
| + | &=& \left(-h\alpha\vec{\jmath}_0+\dfrac{h^2\Omega^2}{R}\vec{k}_0\right)+ | ||
| + | \left(-\Omega^2h\vec{\imath}_0+\alpha h\vec{\jmath}_0\right)+2\left(\Omega\vec{k}_0\right)\times(-\Omega h\vec{\jmath}_0)=\\ | ||
| + | &=&h\Omega^2\vec{\imath}_0+\dfrac{h^2\Omega^2}{R}\vec{k}_0\end{array}</math></center> | ||
| + | |||
| + | Para el D | ||
| + | |||
| + | <center><math>\begin{array}{rcl}\vec{a}^D_{21}&=&\vec{a}^D_{20}+\vec{a}^D_{01}+2\vec{\omega}_{01}\times\vec{v}^D_{20}=\\ | ||
| + | &=& \left(h\alpha\vec{\jmath}_0-\dfrac{h^2\Omega^2}{R}\vec{k}_0\right)+ | ||
| + | \left(-\Omega^2h\vec{\imath}_0+\alpha h\vec{\jmath}_0\right)+2\left(\Omega\vec{k}_0\right)\times(\Omega h\vec{\jmath}_0)=\\ | ||
| + | &=&-3h\Omega^2\vec{\imath}_0+2h\alpha\vec{\jmath}_0-\dfrac{h^2\Omega^2}{R}\vec{k}_0\end{array}</math></center> | ||
[[Categoría:Problemas de cinemática del movimiento relativo (CMR)]] | [[Categoría:Problemas de cinemática del movimiento relativo (CMR)]] | ||
última version al 15:00 11 ene 2018
Contenido |
1 Enunciado
Un disco de radio R (“sólido 2”) se encuentra ensartado mediante un rodamiento sin fricción en un eje horizontal de longitud h (“sólido 0”). Este eje está montado sobre un soporte vertical fijo de altura R. El disco rueda sin deslizar sobre la superficie horizontal z = 0 (“sólido 1”). Consideramos tres sistemas de referencia. Uno fijo en el suelo, uno ligado al disco, y uno intermedio en el que el eje OX0 es a lo largo de la barra horizontal y OZ0 = OZ1 en todo momento. Sea θ(t) el ángulo que el eje OX0 forma con el OX1. En un instante dado 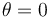 ,
, 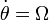 ,
, 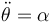 .
.
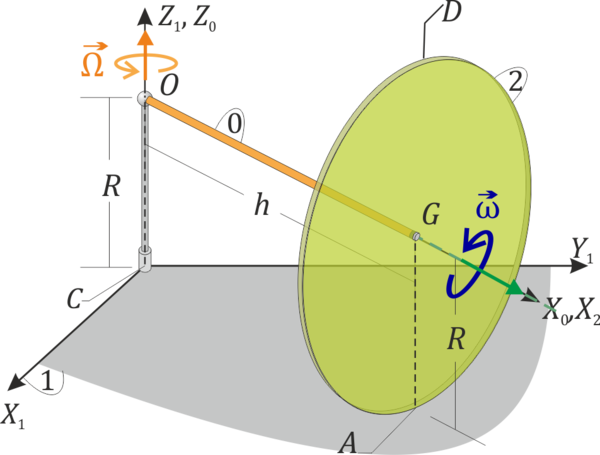
Para ese instante:
- Determine los vectores
 ,
, 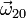 y
y 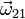 .
.
- Halle la posición de los ejes instantáneos de rotación en los movimientos {01}, {20} y {21}.
- Calcule las velocidades en el movimiento {21} y el {20} del punto A de contacto del disco con el suelo; del G, centro del disco, y de D, el punto más alto del disco.
- Halle las aceleraciones angulares
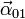 ,
,  y
y 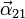 .
.
- Calcule las aceleraciones en los movimientos {21} y {20} de los puntos A, G y D del apartado (3).
2 Velocidades angulares
En este problema tenemos tres sistemas de referencia, los dos sólidos y el sistema intermedio.
Lo primero que observamos es que el punto O es fijo en los tres movimientos: En el {01} porque pertenece al eje de rotación OZ1. En el {20} porque pertenece al eje OX0, de rotación en este movimiento, y en el {21} por composición de velocidades

Puesto que el punto O es fijo en los tres, podemos emplearlo como origen de coordenadas común.
El sistema “1” es el fijo, con ejes OX1 y OY1 horizontales y OZ1 vertical.
El sistema “0” tiene su eje OX0 siempre alineado con la varilla, siendo OZ0 vertical y coincidente con OZ1. En el movimiento {01} este sistema gira en torno a OZ1 = OZ0 por lo que las bases respectivas cumplen
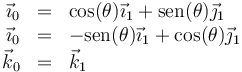
La velocidad angular del movimiento {01} es una rotación en torno a su eje común

El sistema 2 es uno ligado al disco y cuyo eje OX2 coincide con el OX0. El disco gira alrededor de este eje. Por tanto, la relación entre las correspondientes bases es
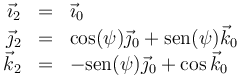
siendo la velocidad angular

Aquí ψ es el ángulo que va girando el disco sobre su eje. No es una variable independiente de θ ya que debe cumplirse la condición de rodadura sin deslizamiento.
La velocidad angular del movimiento {21} es la composición de las otras dos
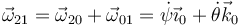
Obtenemos la relación entre las dos componentes imponiendo la condición de que la velocidad en el punto A, de contacto entre el disco y el plano, es nula en el movimiento {21}
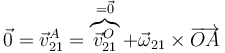
Desarrollando el producto vectorial

por lo que debe ser

y por tanto, si llamamos 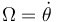

3 Ejes instantáneos de rotación
Lo localización de los tres EIR es sencilla:
- Movimiento {01}
- El eje es el OZ1 = OZ0
- Movimiento {20}
- El eje es el OX2 = OX0
- Movimiento {21}
- Es el que pasa por O y lleva la dirección de
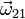 o, lo que es equivalente, el que pasa por O y A, al ser ambos puntos de velocidad nula en el movimiento {01}.
o, lo que es equivalente, el que pasa por O y A, al ser ambos puntos de velocidad nula en el movimiento {01}.
4 Velocidades lineales
4.1 Del punto A
En el movimiento {21}, la velocidad del punto A es nula según hemos dicho.
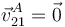
En el movimiento {20} el punto A describe un movimiento circular en torno al eje del disco, que pasa por el origen O.
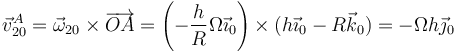
También se puede hallar esta velocidad a partir de la del movimiento {01} ya que por ser nula la del {21}
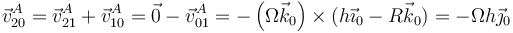
4.2 Del punto G
En el movimiento {20} G está en reposo por pertenecer al eje de rotación
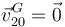
En el {21} y en el {01} realiza un movimiento de rotación alrededor de un eje que pasa por A.

Vemos que en este movimiento A y G tienen la misma velocidad por estar a la misma distancia del eje. De aquí
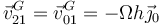
4.3 Del punto D
Operando igualmente, tenemos en el movimiento {01}
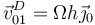
En el {20}
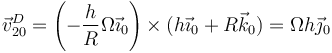
y por tanto
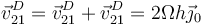
5 Aceleraciones angulares
El movimiento {01} es una rotación con eje fijo, siendo su aceleración angular

Análogamente ocurre para el {20}
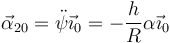
Para el {21} empleamos la composición de aceleraciones angulares
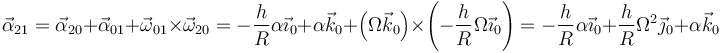
6 Aceleraciones lineales
6.1 En el movimiento {01}
En el movimiento {01} todos los puntos describen un moviento circular acelerado alrededor del eje OZ1. Puesto que los tres puntos A, G y D, se hallan a la misma distancia del eje, la aceleración en este movimiento es igual para todos ellos
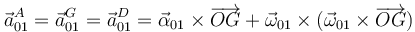
Puesto que este movimiento es plano, podemos simplificar la expresión
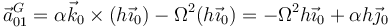
6.2 En el movimiento {20}
Este movimiento es de rotación en torno al eje OX0, el cual pasa por G. Por tanto
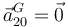
Para el punto A, situado en el mismo plano director que G
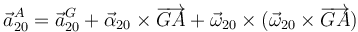
lo que nos da
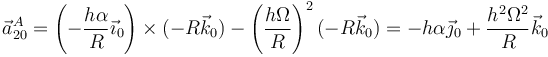
Para el punto D el cálculo es similar, invirtiendo el vector de posición relativa
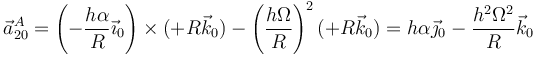
6.3 En el movimiento {21}
Aquí podemos calcularlas directamente con la fórmula análoga
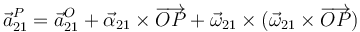
o bien con el teorema de Coriolis
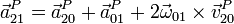
Si empleamos el teorema de Coriolis, para el punto G resulta
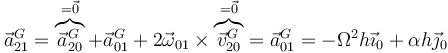
Para el punto A
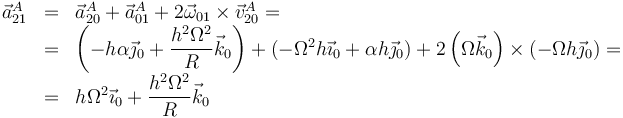
Para el D