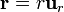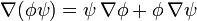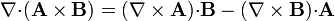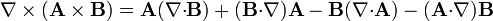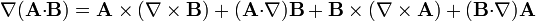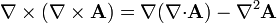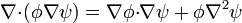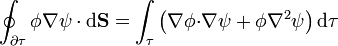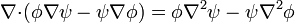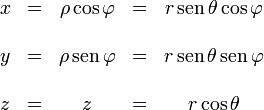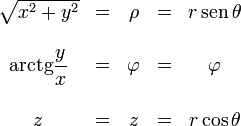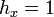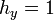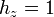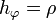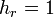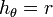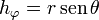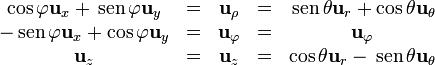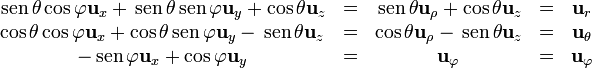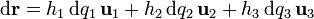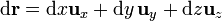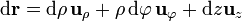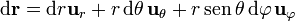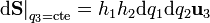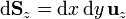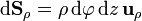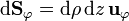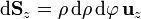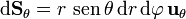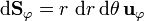Tabla de cálculo vectorial
De Laplace
(Diferencias entre revisiones)
(→Esféricas) |
(→En esféricas) |
||
| (12 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
| - | |||
==Álgebra del operador nabla== | ==Álgebra del operador nabla== | ||
===Aplicación sobre productos=== | ===Aplicación sobre productos=== | ||
| Línea 63: | Línea 62: | ||
<math>\mathbf{r}=r\mathbf{u}_{r}</math> | <math>\mathbf{r}=r\mathbf{u}_{r}</math> | ||
| - | ==[[Factores de escala]]== | + | ==[[Bases vectoriales|Factores de escala]]== |
===Definición=== | ===Definición=== | ||
:<math>h_i=\left|\frac{\partial \mathbf{r}}{\partial q_i}\right|</math> | :<math>h_i=\left|\frac{\partial \mathbf{r}}{\partial q_i}\right|</math> | ||
| Línea 75: | Línea 74: | ||
===Esféricas=== | ===Esféricas=== | ||
:<math>h_r=1\,</math>{{qquad}}<math>h_\theta=r\,</math>{{qquad}}<math>h_\varphi=r\,\operatorname{sen}\,\theta</math> | :<math>h_r=1\,</math>{{qquad}}<math>h_\theta=r\,</math>{{qquad}}<math>h_\varphi=r\,\operatorname{sen}\,\theta</math> | ||
| + | |||
| + | ==Relación entre bases vectoriales== | ||
| + | ===De cartesianas a otro sistema=== | ||
| + | :<math>\begin{array}{ccccc} | ||
| + | \mathbf{u}_{x} & = & \cos\varphi\mathbf{u}_{\rho}-\,\operatorname{sen}\,\varphi\mathbf{u}_{\varphi} & = & | ||
| + | \,\operatorname{sen}\,\theta\cos\varphi\mathbf{u}_{r}+\cos\theta\cos\varphi\mathbf{u}_{\theta}-\,\operatorname{sen}\,\varphi\mathbf{u}_{\varphi} \\ | ||
| + | \mathbf{u}_{y} & = & \,\operatorname{sen}\,\varphi\mathbf{u}_{\rho}+\cos\varphi\mathbf{u}_{\varphi} & = & | ||
| + | \,\operatorname{sen}\,\theta\,\operatorname{sen}\,\varphi\mathbf{u}_{r}+\cos\theta\,\operatorname{sen}\,\varphi\mathbf{u}_{\theta}+\cos\varphi\mathbf{u}_{\varphi} \\ | ||
| + | \mathbf{u}_{z} & = & \mathbf{u}_{z} & = & \cos\theta\mathbf{u}_{r}-\,\operatorname{sen}\,\theta\mathbf{u}_{\theta} | ||
| + | \end{array}</math> | ||
| + | |||
| + | ===De cilíndricas a otro sistema=== | ||
| + | :<math>\begin{array}{ccccc} | ||
| + | \cos\varphi\mathbf{u}_{x}+\,\operatorname{sen}\,\varphi\mathbf{u}_{y} & = & \mathbf{u}_{\rho} & = & | ||
| + | \,\operatorname{sen}\,\theta\mathbf{u}_{r}+\cos\theta\mathbf{u}_{\theta} \\ | ||
| + | -\,\operatorname{sen}\,\varphi\mathbf{u}_{x}+\cos\varphi\mathbf{u}_{y} | ||
| + | & = & | ||
| + | \mathbf{u}_{\varphi}& = & | ||
| + | \mathbf{u}_{\varphi} \\ | ||
| + | \mathbf{u}_{z} & = & \mathbf{u}_{z} & = & \cos\theta\mathbf{u}_{r}-\,\operatorname{sen}\,\theta\mathbf{u}_{\theta}\\ | ||
| + | \end{array}</math> | ||
| + | |||
| + | ===De esféricas a otro sistema=== | ||
| + | :<math>\begin{array}{ccccc} | ||
| + | \,\operatorname{sen}\,\theta\cos\varphi\mathbf{u}_{x}+\,\operatorname{sen}\,\theta\,\operatorname{sen}\,\varphi\mathbf{u}_{y}+\cos\theta\mathbf{u}_{z} & = & \,\operatorname{sen}\,\theta\mathbf{u}_{\rho}+\cos\theta\mathbf{u}_{z} & = & | ||
| + | \mathbf{u}_{r} \\ | ||
| + | \cos\theta\cos\varphi\mathbf{u}_{x}+\cos\theta\,\operatorname{sen}\,\varphi\mathbf{u}_{y}-\,\operatorname{sen}\,\theta\mathbf{u}_{z} & = &\cos\theta\mathbf{u}_{\rho}-\,\operatorname{sen}\,\theta\mathbf{u}_{z} & = & | ||
| + | \mathbf{u}_{\theta} \\ | ||
| + | -\,\operatorname{sen}\,\varphi\mathbf{u}_{x}+\cos\varphi\mathbf{u}_{y} | ||
| + | & = & | ||
| + | \mathbf{u}_{\varphi}& = & | ||
| + | \mathbf{u}_{\varphi} \\ | ||
| + | \end{array}</math> | ||
| + | |||
| + | ==Diferenciales== | ||
| + | ===De camino=== | ||
| + | ====Para coordenadas ortogonales==== | ||
| + | :<math>\mathrm{d}\mathbf{r}=h_1\,\mathrm{d}q_1\,\mathbf{u}_{1}+h_2\,\mathrm{d}q_2\,\mathbf{u}_{2}+h_3\,\mathrm{d}q_3\,\mathbf{u}_{3}</math> | ||
| + | |||
| + | ====En cartesianas==== | ||
| + | :<math>\mathrm{d}\mathbf{r}=\mathrm{d}x\mathbf{u}_{x}+\mathrm{d}y\,\mathbf{u}_{y}+\mathrm{d}z\mathbf{u}_{z}</math> | ||
| + | ====En cilíndricas==== | ||
| + | :<math>\mathrm{d}\mathbf{r}=\mathrm{d}\rho\,\mathbf{u}_{\rho}+\rho\,\mathrm{d}\varphi\,\mathbf{u}_{\varphi}+\mathrm{d}z\mathbf{u}_{z}</math> | ||
| + | ====En esféricas==== | ||
| + | :<math>\mathrm{d}\mathbf{r}=\mathrm{d}r\mathbf{u}_{r}+r\,\mathrm{d}\theta\,\mathbf{u}_{\theta}+r\,\operatorname{sen}\,\theta\,\mathrm{d}\varphi\,\mathbf{u}_{\varphi}</math> | ||
| + | |||
| + | ===De superficie=== | ||
| + | ====Para coordenadas ortogonales==== | ||
| + | :<math>\left.\mathrm{d}\mathbf{S}\right|_{q_3=\mathrm{cte}}= h_1 h_2 \mathrm{d}q_1 \mathrm{d}q_2 \mathbf{u}_{3}</math> | ||
| + | ====En cartesianas==== | ||
| + | :<math>\mathrm{d}\mathbf{S}_x=\mathrm{d}y\,\mathrm{d}z\,\mathbf{u}_{x}</math> | ||
| + | :<math>\mathrm{d}\mathbf{S}_y=\mathrm{d}x\,\mathrm{d}z\,\mathbf{u}_{y}</math> | ||
| + | :<math>\mathrm{d}\mathbf{S}_z=\mathrm{d}x\,\mathrm{d}y\,\mathbf{u}_{z}</math> | ||
| + | |||
| + | ====En cilíndricas==== | ||
| + | :<math>\mathrm{d}\mathbf{S}_\rho=\rho\,\mathrm{d}\varphi\,\mathrm{d}z\,\mathbf{u}_{\rho}</math> | ||
| + | :<math>\mathrm{d}\mathbf{S}_\varphi=\mathrm{d}\rho\,\mathrm{d}z\,\mathbf{u}_{\varphi}</math> | ||
| + | :<math>\mathrm{d}\mathbf{S}_z=\rho\,\mathrm{d}\rho\,\mathrm{d}\varphi\,\mathbf{u}_{z}</math> | ||
| + | |||
| + | ====En esféricas==== | ||
| + | :<math>\mathrm{d}\mathbf{S}_r=r^2\,\,\operatorname{sen}\,\theta\,\mathrm{d}\theta\,\mathrm{d}\varphi\,\mathbf{u}_{r}</math> | ||
| + | :<math>\mathrm{d}\mathbf{S}_\theta=r\,\,\operatorname{sen}\,\theta\,\mathrm{d}r\,\mathrm{d}\varphi\,\mathbf{u}_{\theta}</math> | ||
| + | :<math>\mathrm{d}\mathbf{S}_\varphi=r\,\,\mathrm{d}r\,\mathrm{d}\theta\,\mathbf{u}_{\varphi}</math> | ||
| + | |||
| + | ===De volumen=== | ||
| + | ====Para coordenadas ortogonales==== | ||
| + | ====En cartesianas==== | ||
| + | ====En cilíndricas==== | ||
| + | ====En esféricas==== | ||
última version al 11:52 25 jul 2008
Contenido[ocultar]
|
1 Álgebra del operador nabla
1.1 Aplicación sobre productos
1.1.1 De dos campos escalares
1.1.2 De un campo escalar por uno vectorial
1.1.3 De dos campos vectoriales
1.2 Operadores de segundo orden
1.3 Identidades de Green
1.3.1 Primera
1.3.1.1 En forma diferencial
1.3.1.2 En forma integral
1.3.2 Segunda
1.3.2.1 En forma diferencial
1.3.2.2 En forma integral
2 Relación entre los sistemas de coordenadas
2.1 De cartesianas a otros sistemas
2.2 De cilíndricas a otros sistemas
2.3 De esféricas a otros sistemas
3 Vector de posición
3.1 En cartesianas
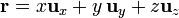
3.2 En cilíndricas
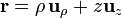
3.3 En esféricas