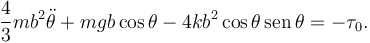Dos barras articuladas con muelle (Feb. 2020)
De Laplace
(→Ecuaciones de Lagrange) |
|||
| (2 ediciones intermedias no se muestran.) | |||
| Línea 125: | Línea 125: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | U^{'2'}_g = | + | U^{'2'}_g = mbg\,\mathrm{sen}\,\theta. |
</math> | </math> | ||
</center> | </center> | ||
| Línea 137: | Línea 137: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | U = U_g + U_k = | + | U = U_g + U_k = mbg\,\mathrm{sen}\,\theta + 2kb^2\cos^2\theta. |
</math> | </math> | ||
</center> | </center> | ||
| Línea 146: | Línea 146: | ||
<math> | <math> | ||
\dfrac{\partial U}{\partial\theta} = | \dfrac{\partial U}{\partial\theta} = | ||
| - | + | mgb\cos\theta - 4kb^2\,\mathrm{sen}\,\theta\cos\theta = | |
0 | 0 | ||
\Longrightarrow | \Longrightarrow | ||
| - | \cos\theta\,(mg- | + | \cos\theta\,(mg-4kb\,\mathrm{sen}\,\theta) = 0. |
</math> | </math> | ||
</center> | </center> | ||
| Línea 158: | Línea 158: | ||
\cos\theta = 0 \to \left\{\begin{array}{l} \theta=\pi/2 \\ \theta=-\pi/2\end{array}\right.\\ | \cos\theta = 0 \to \left\{\begin{array}{l} \theta=\pi/2 \\ \theta=-\pi/2\end{array}\right.\\ | ||
\\ | \\ | ||
| - | \mathrm{sen}\,\theta = mg/ | + | \mathrm{sen}\,\theta = mg/4kb. |
\end{array} | \end{array} | ||
</math> | </math> | ||
</center> | </center> | ||
| - | La última posición sólo puede observarse si <math>mg< | + | La última posición sólo puede observarse si <math>mg<4kb</math>, pues el seno debe ser siempre menor que 1. |
Aunque no se pide en el enunciado, vamos a analizar la estabilidad de estas posiciones de equilibrio. Para ello calculamos la derivada segunda del potencial | Aunque no se pide en el enunciado, vamos a analizar la estabilidad de estas posiciones de equilibrio. Para ello calculamos la derivada segunda del potencial | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \dfrac{\ | + | \dfrac{\partial^2U}{\partial\theta^2} = -mgb\,\mathrm{sen}\,\theta - 4kb^2\,(1-2\,\mathrm{sen}^2\,\theta) |
</math> | </math> | ||
</center> | </center> | ||
| Línea 174: | Línea 174: | ||
<math> | <math> | ||
\begin{array}{lclcl} | \begin{array}{lclcl} | ||
| - | \theta = \pi/2 & \to & \dfrac{\ | + | \theta = \pi/2 & \to & \dfrac{\partial^2U}{\partial\theta^2} = 4kb^2-mgb &\to &\left\{ |
\begin{array}{l} | \begin{array}{l} | ||
| - | + | 4kb>mg \to \mathrm{Minimo} \to \mathrm{Estable}\\ | |
| - | + | 4kb<mg \to \mathrm{Maximo} \to \mathrm{Inestable}\\ | |
| - | + | 4kb=mg \to \mathrm{Inflexion} \to \mathrm{Indiferente} | |
\end{array} | \end{array} | ||
\right. | \right. | ||
\\ | \\ | ||
| - | \theta = -\pi/2 & \to & \dfrac{\ | + | \theta = -\pi/2 & \to & \dfrac{\partial^2U}{\partial\theta^2} = mgb + 4kb^2&\to & \mathrm{Minimo} \to \mathrm{Estable}\\ |
| - | \mathrm{sen}\,\theta = mg/ | + | \mathrm{sen}\,\theta = mg/4kb & \to & \dfrac{\partial^2U}{\partial\theta^2} = -4kb^2+ m^2g^2/4k &\to &\left\{ |
\begin{array}{l} | \begin{array}{l} | ||
| - | + | 4kb>mg \to \mathrm{Maximo} \to \mathrm{Inestable}\\ | |
| - | + | 4kb<mg \to \mathrm{No\, aparece}\\ | |
| - | + | 4kb=mg \to \mathrm{Inflexion} \to \mathrm{Indiferente} | |
\end{array} | \end{array} | ||
\right. | \right. | ||
| Línea 194: | Línea 194: | ||
</center> | </center> | ||
[[Archivo:MRGIC-BarrasArticuladas-Curvas.png|right]] | [[Archivo:MRGIC-BarrasArticuladas-Curvas.png|right]] | ||
| - | La gráfica representa la función <math>U/kb^2</math> en función del ángulo. Vemos que cuando <math>mg< | + | La gráfica representa la función <math>U/kb^2</math> en función del ángulo. Vemos que cuando <math>mg<4kb</math> la función tiene dos mínimos y un máximo. Los mínimos son las soluciones estables (<math>\theta=\pm\pi/2</math>) y el máximo la inestable (<math>\mathrm{sen}\,\theta=mg/4kb</math>). |
| - | Cuando <math>mg\ | + | Cuando <math>mg\geq4kb</math> sólo hay un mínimo (<math>\theta=-\pi/2</math>). En el caso <math>mg=4kb</math> la curva es plana en <math>\theta=\pi/2</math> correspondiendo a la situación de equilibrio indiferente. |
== Diagrama de fuerzas con par aplicado == | == Diagrama de fuerzas con par aplicado == | ||
| Línea 338: | Línea 338: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | L = T - U = \dfrac{2}{3}mb^2\dot{\theta}^2 - | + | L = T - U = \dfrac{2}{3}mb^2\dot{\theta}^2 - mgb\,\mathrm{sen}\,\theta - 2kb^2\cos\theta^2. |
</math> | </math> | ||
</center> | </center> | ||
| Línea 359: | Línea 359: | ||
\\ | \\ | ||
\dfrac{\partial L}{\partial\theta} = | \dfrac{\partial L}{\partial\theta} = | ||
| - | - | + | -mgb\cos\theta + 4kb^2\cos\theta\,\mathrm{sen}\,\theta.\\ |
\\ | \\ | ||
Q^{\tau}_{\theta} = \vec{\tau}\cdot\dfrac{\partial\vec{\omega}_{21}}{\partial\dot{\theta}} | Q^{\tau}_{\theta} = \vec{\tau}\cdot\dfrac{\partial\vec{\omega}_{21}}{\partial\dot{\theta}} | ||
| Línea 370: | Línea 370: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \dfrac{4}{3}mb^2\ddot{\theta} + | + | \dfrac{4}{3}mb^2\ddot{\theta} + mgb\cos\theta - 4kb^2\cos\theta\,\mathrm{sen}\,\theta = -\tau_0. |
</math> | </math> | ||
</center> | </center> | ||
[[Categoría:Problemas de examen de Mecánica Racional]] | [[Categoría:Problemas de examen de Mecánica Racional]] | ||
última version al 19:15 9 feb 2021
Contenido |
1 Enunciado
El sistema de la figura consta de dos barra articuladas. La longitud de las dos barras es L = 2b. La masa de la barra "2" es m, mientras que la de la masa "0" es despreciable. Las barras se articulan entre sí en el punto B. El extremo A de la barra "0" se conecta con un pasador, de modo que desliza sobre el eje fijo OX1. La barra "2" está articulada sobre el eje OX1 en el punto fijo C. Un muelle de constante elástica k y longitud natural nula conecta los centros de las barras.
- Determina gráfica y analíticamente la posición de los CIR de los tres movimientos que se pueden definir en el sistema.
- ¿Cuántos grados de libertad tiene el problema?. Encuentra reducciones cinemáticas de los movimientos {21}, {20} y {01}. Las reducciones deben expresarse en función de los grados de libertad.
- Calcula la energía cinética y potencial del sistema.
- Determina la posición de equilibrio.
- Se aplica un par
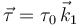 sobre la barra "2". Dibuja el diagrama de fuerzas y pares que actúan sobre cada barra.
sobre la barra "2". Dibuja el diagrama de fuerzas y pares que actúan sobre cada barra.
- Calcula la cantidad de movimiento de cada barra.
- Calcula
 y
y  .
.
- Aplicando el T.C.M. y el T.M.C. encuentra las ecuaciones que describen el sistema.
- Escribe la función de Lagrange y las ecuaciones de Lagrange del sistema.
2 Solución
2.1 Posiciones de los C.I.R.s
Las barras están articuladas en el punto B. Por tanto
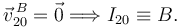
La barra "2" esta articulada en el punto fijo C. Entonces
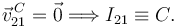
El punto A desliza sobre el eje fijo OX1. Entonces 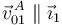 . El C.I.R. I01 debe estar en la recta perpendicular a esta velocidad trazada en A. Por otro lado, el Teorema de los Tres Centros nos dice que ese C.I.R. también debe encontrarse en la recta que une I21 y I20. El punto de corte nos da la posición de I01. Los vectores de posición de estos puntos son
. El C.I.R. I01 debe estar en la recta perpendicular a esta velocidad trazada en A. Por otro lado, el Teorema de los Tres Centros nos dice que ese C.I.R. también debe encontrarse en la recta que une I21 y I20. El punto de corte nos da la posición de I01. Los vectores de posición de estos puntos son
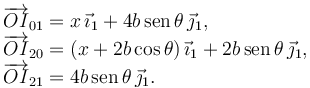
2.2 Cinemática
Observando el dibujo tenemos

Para la barra "2" tenemos
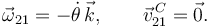
Ahora aplicamos el vínculo en el punto de articulación de las barras:  . Usando las leyes de composición de velocidades
. Usando las leyes de composición de velocidades

Para que esa velocidad se anula debe cumplirse
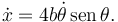
Otra forma de obtener esta expresión es darse cuenta de que

Derivando respecto al tiempo esta expresión se obtiene el resultado anterior.
Así pues, el sistema tiene un grado de libertad. Elegimos la coordenada {θ} para trabajar. Las reducciones cinemáticas quedan

2.3 Energía cinética y potencial
La masa de la barra "0" es despreciable. Por tanto, no contribuye ni a la energía cinética ni a la energía potencial del sistema.
La barra "2" tiene un punto fijo. Entonces su energía cinética puede expresarse

Su energía potencial gravitatoria es
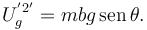
La energía potencial elástica del muelle es
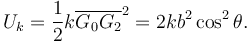
Entonces, la energía potencial total es
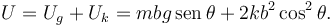
2.4 Posición de equilibrio
Como no hay fuerzas aplicadas no conservativas, la condición de equilibrio es
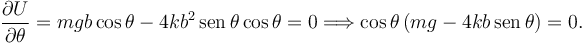
Tenemos dos posibles soluciones
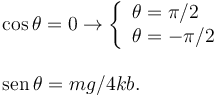
La última posición sólo puede observarse si mg < 4kb, pues el seno debe ser siempre menor que 1.
Aunque no se pide en el enunciado, vamos a analizar la estabilidad de estas posiciones de equilibrio. Para ello calculamos la derivada segunda del potencial
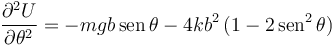
Ahora evaluamos la segunda derivada en las posiciones de equilibrio
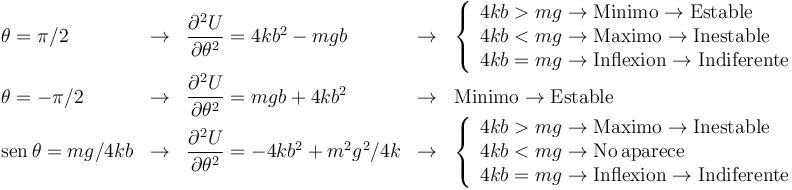
La gráfica representa la función U / kb2 en función del ángulo. Vemos que cuando mg < 4kb la función tiene dos mínimos y un máximo. Los mínimos son las soluciones estables (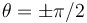 ) y el máximo la inestable (
) y el máximo la inestable (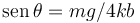 ).
Cuando
).
Cuando 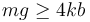 sólo hay un mínimo (θ = − π / 2). En el caso mg = 4kb la curva es plana en θ = π / 2 correspondiendo a la situación de equilibrio indiferente.
sólo hay un mínimo (θ = − π / 2). En el caso mg = 4kb la curva es plana en θ = π / 2 correspondiendo a la situación de equilibrio indiferente.
2.5 Diagrama de fuerzas con par aplicado
La figura de la derecha muestra las fuerzas y pares que actúan sobre cada sólido. Sus expresiones en la base "1" son
Sólido 2
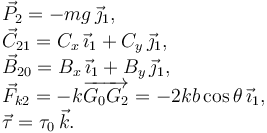
Sólido 0
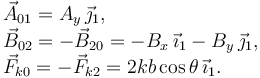
Las incógnitas del problema son: {θ,Cx,Cy,Bx,By,Ay}. Son 6, por lo que el problema puede resolverse aplicando los teoremas fundamentales.
2.6 Cantidad de movimiento y momentos angulares
La barra "0" no tiene ni cantidad de movimiento ni momento angular, pues no tiene masa. Para la barra "2" la cantidad de movimiento es
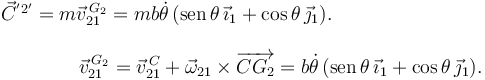
Para calcular el momento angular pedido aprovechamos que C es un punto fijo
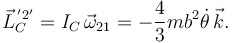
2.7 Ecuaciones aplicando los teoremas fundamentales
2.7.1 T.C.M.
Sólido 2 Tenemos
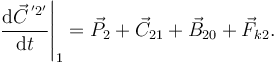
La derivada temporal es
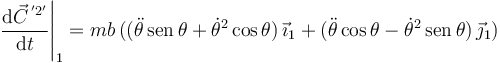
Obtenemos así dos ecuaciones
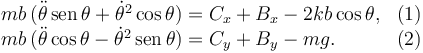
Sólido 2 En este caso la derivada temporal se anula pues la cantidad de movimiento es cero
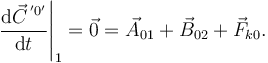
Obtenemos así dos ecuaciones
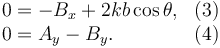
2.7.2 T.M.C.
Sólido 2 Como el punto C es fijo, aplicamos el T.M.C. en él
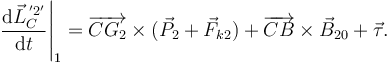
El peso y la fuerza elástica están aplicados en el mismo punto y el par externo está aplicado en el sólido "2".
La derivada temporal es
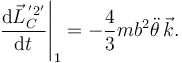
Haciendo los productos vectoriales (sabiendo que 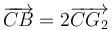 obtenemos otra ecuación
obtenemos otra ecuación
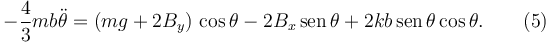
Sólido 0 De nuevo, la derivada temporal es nula porque el momento angular es nulo. Aplicando el T.M.C. en el centro de masas tenemos
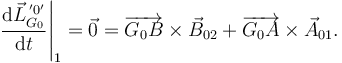
Haciendo los productos vectoriales obtenemos la última ecuación
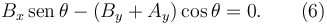
2.8 Ecuaciones de Lagrange
La función de Lagrange es
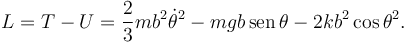
Hay sólo una ecuación de Lagrange, pues hay sólo un grado de libertad. Hay que tener en cuenta que hay un par aplicado que no está incluido en la Lagrangiana. Por tanto, la ecuación es
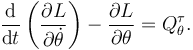
Calculamos los diferentes términos

Finalmente, la ecuación de Lagrange es