No Boletín - Cuestión sobre EIRMD III (Ex.Ene/16)
De Laplace
Contenido |
1 Enunciado
Un sólido rígido realiza un movimiento helicoidal instantáneo respecto a un triedro de referencia 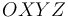 , estando
definido su campo de velocidades mediante la siguiente reducción cinemática en el origen de coordenadas
, estando
definido su campo de velocidades mediante la siguiente reducción cinemática en el origen de coordenadas  :
:

- Calcule la velocidad de deslizamiento del sólido rígido (segundo invariante).
- ¿Por cuál de los siguientes puntos pasa el eje instantáneo de rotación y mínimo deslizamiento?

2 Velocidad de deslizamiento
La velocidad de deslizamiento  (segundo invariante) es la proyección de la velocidad de cualquier punto sobre la velocidad angular:
(segundo invariante) es la proyección de la velocidad de cualquier punto sobre la velocidad angular:
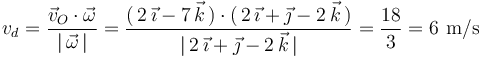
3 Punto perteneciente al EIRMD. Primer método: cálculo de la velocidad del punto 
Utilizando la ecuación del campo de velocidades del sólido rígido, calculamos la velocidad 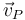 del punto
del punto 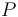 en cada una de las opciones:
en cada una de las opciones:
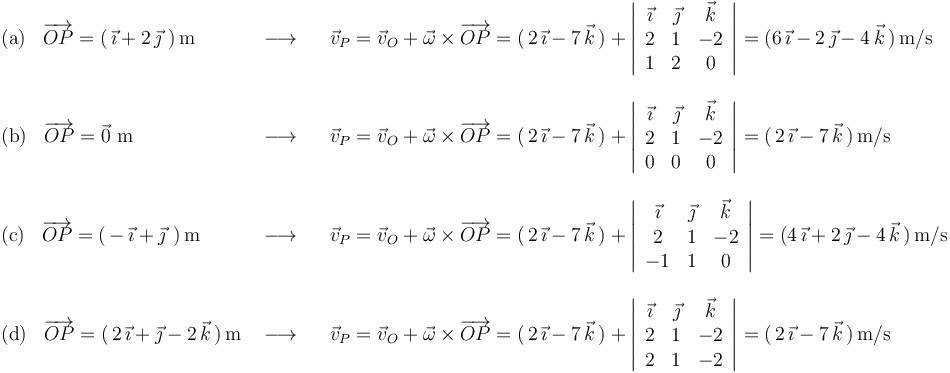
Si el punto 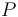 pertenece al eje instantáneo de rotación y mínimo deslizamiento (EIRMD), la velocidad
pertenece al eje instantáneo de rotación y mínimo deslizamiento (EIRMD), la velocidad 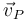 de dicho punto es necesariamente paralela al vector velocidad angular
de dicho punto es necesariamente paralela al vector velocidad angular 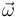 . Comprobamos que tal cosa sólo ocurre en la opción (c), la cual es por tanto la respuesta correcta:
. Comprobamos que tal cosa sólo ocurre en la opción (c), la cual es por tanto la respuesta correcta:
4 Punto perteneciente al EIRMD. Segundo método: determinación del EIRMD
Partiendo del conocimiento de la reducción cinemática  , es posible determinar el EIRMD del movimiento helicoidal instantáneo. En efecto: aplicando la ecuación vectorial del EIRMD, obtenemos el vector de posición de un punto genérico
, es posible determinar el EIRMD del movimiento helicoidal instantáneo. En efecto: aplicando la ecuación vectorial del EIRMD, obtenemos el vector de posición de un punto genérico  del EIRMD:
del EIRMD:
![\overrightarrow{OI}=\frac{\vec{\omega}\times\vec{v}_O}{|\,\vec{\omega}\,|^2}\,+\,\lambda\,\vec{\omega}=\frac{1}{9}\left|\begin{array}{ccc} \vec{\imath} & \vec{\jmath} & \vec{k} \\ 2 & 1 & -2 \\ 2 & 0 & -7 \end{array}\right|\,+\,\lambda\,(2\,\vec{\imath}\,\,+\,\vec{\jmath}\,-\,2\,\vec{k}\,)=\left[\,\left(-\frac{7}{9}\,+\,2\lambda\right)\vec{\imath}\,+\left(\frac{10}{9}\,+\,\lambda\right)\vec{\jmath}\,+\left(-\frac{2}{9}\,-\,2\lambda\right)\vec{k}\,\right]\,\mathrm{m}](/wiki/images/math/1/d/b/1dbe9bb4a9ad9b692794c3dfb4b0c6d0.png)
Por tanto, las coordenadas de un punto genérico 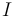 del EIRMD en el triedro OXYZ de referencia son:
del EIRMD en el triedro OXYZ de referencia son:
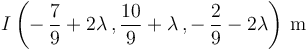
Comparando esta terna  paramétrica de coordenadas con las ternas de los cuatro puntos
paramétrica de coordenadas con las ternas de los cuatro puntos  propuestos en el enunciado, deducimos que el único punto
propuestos en el enunciado, deducimos que el único punto  es el de la opción (c). En efecto:
es el de la opción (c). En efecto: 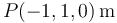 es el punto del EIRMD correspondiente a
es el punto del EIRMD correspondiente a  .
.





