No Boletín - Area de un polígono (Ex.Oct/17)
De Laplace
1 Enunciado
Sea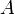 
el área del polígono 
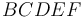de la figura adjunta.
¿Cuál de las siguientes igualdades es falsa?
- (1)
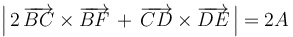
- (2)
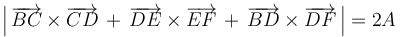
- (3)
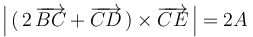
- (4)
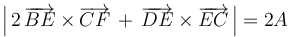
2 Solución
Un detalle importante que conviene observar en las cuatro igualdades propuestas (aplíquese en la (3) la propiedad distributiva respecto a la suma) es que todos los productos vectoriales que aparecen en ellas tienen la misma dirección (perpendicular al plano del polígono) y el mismo sentido (saliente). Nótese que, sólo cuando se suman vectores que tienen la misma dirección y el mismo sentido, se puede igualar el módulo de la suma con la suma de los módulos. Así pues, podemos reescribir las cuatro igualdades propuestas del siguiente modo:
- (1)
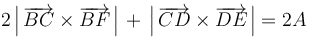
- (2)
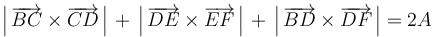
- (3)
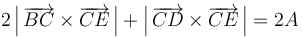
- (4)
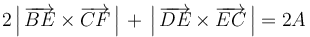
A continuación, interpretamos los términos de estas igualdades recordando la propiedad geométrica del producto vectorial que dice que "el módulo del producto vectorial de dos vectores es igual al área del paralelogramo que tiene a ambos vectores como lados", o bien, que "el módulo del producto vectorial de dos vectores es igual al doble del área del triángulo que tiene a ambos vectores como dos de sus lados".
![\mathrm{(1)}\,\,\,\longrightarrow\,\,\,\underbrace{\underbrace{\underbrace{2\left|\,\overrightarrow{BC}\times\overrightarrow{BF}\,\right|}_{2\,\mathrm{Area}(BCEF)}\,+\,\underbrace{\left|\,\overrightarrow{CD}\times\overrightarrow{DE}\,\right|}_{2\,\mathrm{Area}(CDE)}}_{2\,[\mathrm{Area}(BCEF)\,+\,\mathrm{Area}(CDE)]}}_{2\,\mathrm{Area}(BCDEF)}=2A\,](/wiki/images/math/c/2/c/c2c3b9e22c3bd9482c9912c162631271.png)
![\mathrm{(2)}\,\,\,\longrightarrow\,\,\,\underbrace{\underbrace{\underbrace{\left|\,\overrightarrow{BC}\times\overrightarrow{CD}\,\right|}_{2\,\mathrm{Area}(BCD)}\,+\,\underbrace{\left|\,\overrightarrow{DE}\times\overrightarrow{EF}\,\right|}_{2\,\mathrm{Area}(DEF)}\,+\,\underbrace{\left|\,\overrightarrow{BD}\times\overrightarrow{DF}\,\right|}_{2\,\mathrm{Area}(BDF)}}_{2\,[\mathrm{Area}(BCD)\,+\,\mathrm{Area}(DEF)+\,\mathrm{Area}(BDF)]}}_{2\,\mathrm{Area}(BCDEF)}=2A\,](/wiki/images/math/5/2/5/525c6a2e899862e1ef846f9a6d30fb7b.png)
![\mathrm{(3)}\,\,\,\longrightarrow\,\,\,\underbrace{\underbrace{\underbrace{2\left|\,\overrightarrow{BC}\times\overrightarrow{CE}\,\right|}_{2\,\mathrm{Area}(BCEF)}\,+\,\underbrace{\left|\,\overrightarrow{CD}\times\overrightarrow{CE}\,\right|}_{2\,\mathrm{Area}(CDE)}}_{2\,[\mathrm{Area}(BCEF)\,+\,\mathrm{Area}(CDE)]}}_{2\,\mathrm{Area}(BCDEF)}=2A\,](/wiki/images/math/1/2/b/12b8bb408cc6bbff0d66548ab06620d0.png)
Llegados a este punto, ya hemos comprobado que las igualdades (1), (2) y (3) son correctas. Por tanto, deducimos por eliminación que la igualdad (4) es la que es FALSA.
Si deseamos comprobar que la igualdad (4) es falsa, conviene transformar previamente su primer término hasta obtener productos vectoriales cuyos módulos tengan interpretación geométrica directa:
Y sustituyendo en (4), comprobamos la falsedad de dicha igualdad:
![\mathrm{(4)}\,\,\,\longrightarrow\,\,\,2\left|\,\overrightarrow{BE}\times\overrightarrow{CF}\,\right|\,+\,\left|\,\overrightarrow{DE}\times\overrightarrow{EC}\,\right|=\underbrace{\underbrace{\underbrace{2\left|\,\overrightarrow{BC}\times\overrightarrow{CE}\,\right|}_{2\,\mathrm{Area}(BCEF)}\,+\,\underbrace{2\left|\,\overrightarrow{CE}\times\overrightarrow{EF}\,\right|}_{2\,\mathrm{Area}(BCEF)}\,+\,\underbrace{\left|\,\overrightarrow{DE}\times\overrightarrow{EC}\,\right|}_{2\,\mathrm{Area}(CDE)}}_{2\,\mathrm{Area}(BCEF)\,+\,2\,[\mathrm{Area}(BCEF)+\,\mathrm{Area}(CDE)]}}_{2\,\mathrm{Area}(BCEF)\,+\,2\,\mathrm{Area}(BCDEF)}=2\,\mathrm{Area}(BCEF)\,+\,2A\neq 2A\,](/wiki/images/math/c/a/f/caf99ab61ba5733b6ad15970a848bedb.png)
