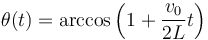De Laplace

Contenido

1 Enunciado
2 Solución

1 Enunciado

Las partículas $A$ y $B$ , ambas con masa $m$ , están unidas por una barra rígida de longitud $2 L$ y masa despreciable. El punto $C$ es el punto medio de la barra. La partícula $A$ está obligada a moverse en el eje fijo $O X$ , como se indica en la figura. Este contacto es liso. La barra que une las partículas forma un ángulo $θ(t)$ con el eje $O X$ . La partícula $A$ se mueve con velocidad constante $\vec{v}_0 = v_0\,\vec{\imath}$ . En el instante inicial la partícula $A$ se encontraba en el punto $O$ y $θ(0) = 0$ . El sistema está sometido a la acción de la gravedad.

Encuentra la expresión de los vectores de posición 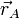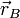$\vec{r}_A$ , $\vec{r}_B$ y $\vec{r}_C$ en función de $v 0$ , $L$ , $θ$ y $t$ .
Si el ángulo varía como 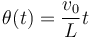$\theta(t)=\dfrac{v_0}{L}t$ , calcula la velocidad y aceleración de las partículas $A$ y $B$ y del centro de masas del sistema.
El movimiento descrito anteriormente está producido por una fuerza horizontal 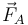$\vec{F}_A$ aplicada sobre la partícula $A$ . Dibuja el diagrama de fuerzas del sistema y calcula la expresión de todas las fuerzas externas que actúan sobre él.
Calcula la energía cinética del sistema y su momento cinético respecto de $O$ en el instante $t 1 = π L / 2 v 0$ .
Supongamos ahora que la partícula $B$ se mueve de modo que la componente de su velocidad sobre el $O X$ es constante e igual a $2 v 0$ . Encuentra y resuelve la ecuación diferencial que debe cumplir $θ(t)$ para que esto sea posible.

2 Solución

2.1 Vectores de posición

Como la partícula $A$ se mueve siempre sobre el eje $O X$ con velocidad uniforme $v 0$ , y empieza su movimiento en el origen tenemos

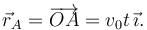
$\vec{r}_A = \overrightarrow{OA} = v_0t\,\vec{\imath}.$

El vector de posición del punto $B$ es

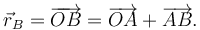
$\vec{r}_B = \overrightarrow{OB} = \overrightarrow{OA} + \overrightarrow{AB}.$

Del dibujo vemos que

$\overrightarrow{AB} = 2L\cos\theta\,\vec{\imath} + 2L\,\mathrm{sen}\,\theta\,\vec{\jmath}.$

Entonces

$\vec{r}_B = \overrightarrow{OB} = (v_0t + 2L\cos\theta)\,\vec{\imath} + 2L\,\mathrm{sen}\,\theta\,\vec{\jmath}$

Para el punto $C$ tenemos

$\vec{r}_C = \overrightarrow{OC} = \overrightarrow{OA} + \overrightarrow{AC} = \overrightarrow{OA} + \dfrac{1}{2}\,\overrightarrow{AB} = (v_0t + L\cos\theta)\,\vec{\imath} + L\,\mathrm{sen}\,\theta\,\vec{\jmath}$

2.2 Velocidad y aceleración con $θ(t)$ dada

En este caso tenemos

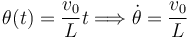
$\theta(t) = \dfrac{v_0}{L}t \Longrightarrow \dot{\theta} = \dfrac{v_0}{L}$

Las velocidades de las partículas $A$ y $B$ son

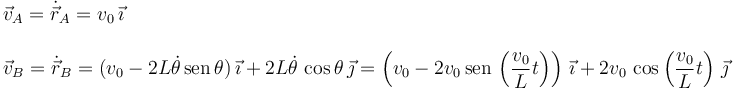
$\begin{array}{l} \vec{v}_A = \dot{\vec{r}}_A = v_0\,\vec{\imath}\\ \\ \vec{v}_B = \dot{\vec{r}}_B = (v_0 -2L\dot{\theta}\,\mathrm{sen}\,\theta)\,\vec{\imath} + 2L\dot{\theta}\,\cos\theta\,\vec{\jmath} = \left(v_0 -2v_0\,\mathrm{sen}\,\left(\dfrac{v_0}{L}t\right)\right)\,\vec{\imath} + 2v_0\,\cos\left(\dfrac{v_0}{L}t\right)\,\vec{\jmath} \end{array}$

La barra no tiene masa. Como las dos masas son iguales, por simetría el Centro de Masas coincide con el punto $C$ . Su velocidad es

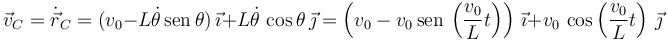
$\vec{v}_C = \dot{\vec{r}}_C = (v_0 -L\dot{\theta}\,\mathrm{sen}\,\theta)\,\vec{\imath} + L\dot{\theta}\,\cos\theta\,\vec{\jmath} = \left(v_0 - v_0\,\mathrm{sen}\,\left(\dfrac{v_0}{L}t\right)\right)\,\vec{\imath} + v_0\,\cos\left(\dfrac{v_0}{L}t\right)\,\vec{\jmath}$

Derivamos otra vez respecto del tiempo para obtener las aceleraciones

$\begin{array}{l} \vec{a}_A = \dot{\vec{v}}_A = \vec{0}\\ \\ \vec{a}_B = \dot{\vec{v}}_B = -2v_0\dot{\theta}\cos\theta\,\vec{\imath} - 2v_0\dot{\theta}\,\mathrm{sen}\,\vec{\jmath} = -\left(\dfrac{2v_0^2}{L}\right)\cos\left(\dfrac{v_0}{L}t\right)\,\vec{\imath} - \left(\dfrac{2v_0^2}{L}\right)\,\mathrm{sen}\,\left(\dfrac{v_0}{L}t\right)\,\vec{\jmath} \\ \\ \vec{a}_C = \dot{\vec{v}}_C = -v_0\dot{\theta}\cos\theta\,\vec{\imath} - v_0\dot{\theta}\,\mathrm{sen}\,\vec{\jmath} = -\left(\dfrac{v_0^2}{L}\right)\cos\left(\dfrac{v_0}{L}t\right)\,\vec{\imath} - \left(\dfrac{v_0^2}{L}\right)\,\mathrm{sen}\,\left(\dfrac{v_0}{L}t\right)\,\vec{\jmath} \end{array}$

2.3 Fuerzas sobre el sistema

La imagen muestra las fuerzas externas que actúan sobre el sistema. Las fuerzas entre la barra y las partículas son internas y no afectan al movimiento. El Teorema de la Cantidad de Movimiento (T.C.M.) dice

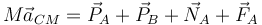
$M\vec{a}_{CM} = \vec{P}_A + \vec{P}_B + \vec{N}_A + \vec{F}_A$

La masa total del sistema es $M = 2 m$ . El Centro de Masas es el punto $C$ . Las fuerzas pueden expresarse como

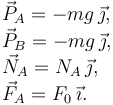
$\begin{array}{l} \vec{P}_A = -mg\,\vec{\jmath},\\ \vec{P}_B = -mg\,\vec{\jmath},\\ \vec{N}_A = N_A\,\vec{\jmath},\\ \vec{F}_A = F_0\,\vec{\imath}. \end{array}$

Las incógnitas son $N A$ y $F 0$ . A partir de T.C.M. tenemos

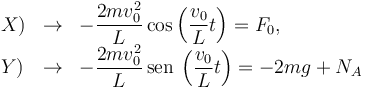
$\begin{array}{lcl} X) & \to & -\dfrac{2mv_0^2}{L}\cos\left(\dfrac{v_0}{L}t\right) = F_0,\\ Y) & \to & -\dfrac{2mv_0^2}{L}\,\mathrm{sen}\,\left(\dfrac{v_0}{L}t\right) = -2mg + N_A\\ \end{array}$

De aquí obtenemos $F 0$ y $N A$ . Tenemos

$\begin{array}{l} \vec{F}_A = -\dfrac{2mv_0^2}{L}\cos\left(\dfrac{v_0}{L}t\right)\,\vec{\imath},\\ \\ \vec{N}_A = \left(2mg - \dfrac{2mv_0^2}{L}\,\mathrm{sen}\,\left(\dfrac{v_0}{L}t\right)\right)\,\vec{\jmath}. \end{array}$

2.4 Energía cinética y momento cinético

En el instante $t 1 = π L / 2 v 0$ tenemos $θ(t 1) = π / 2$ . Entonces los vectores de posición y velocidad de las partículas $A$ y $B$ son

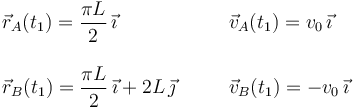
$\begin{array}{lcl} \vec{r}_A(t_1) = \dfrac{\pi L}{2}\,\vec{\imath} & \quad & \vec{v}_A(t_1) = v_0\,\vec{\imath}\\ \\ \vec{r}_B(t_1) = \dfrac{\pi L}{2}\,\vec{\imath} + 2L\,\vec{\jmath} & \quad & \vec{v}_B(t_1) = -v_0\,\vec{\imath} \end{array}$

La energía cinética del sistema es la suma de la energía cinética de las dos partículas (la barra no tiene masa)

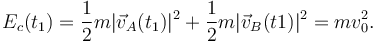
$E_c(t_1) = \dfrac{1}{2}m|\vec{v}_A(t_1)|^2 + \dfrac{1}{2}m|\vec{v}_B(t1)|^2 = mv_0^2.$

El momento cinético del sistema respecto de $O$ es la suma del momento de las dos partículas (de nuevo, la barra no tiene masa)

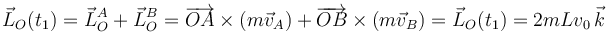
$\vec{L}_O(t_1) = \vec{L}_O^A + \vec{L}_O^B = \overrightarrow{OA}\times(m\vec{v}_A) + \overrightarrow{OB}\times(m\vec{v}_B) = \vec{L}_O(t_1) = 2mLv_0\,\vec{k}$

El primer producto vectorial es cero, pues los dos vectores son paralelos.

2.5 Ecuación diferencial

La velocidad genérica del punto $B$ es

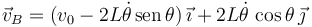
$\vec{v}_B = (v_0 -2L\dot{\theta}\,\mathrm{sen}\,\theta)\,\vec{\imath} + 2L\dot{\theta}\,\cos\theta\,\vec{\jmath}$

La condición del enunciado es

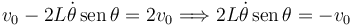
$v_0 -2L\dot{\theta}\,\mathrm{sen}\,\theta = 2v_0 \Longrightarrow 2L\dot{\theta}\,\mathrm{sen}\,\theta = -v_0$

Esta es una ecuación diferencial de variables separables para $θ$ . Tenemos

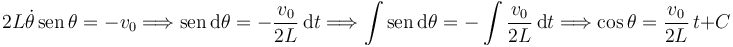
$2L\dot{\theta}\,\mathrm{sen}\,\theta = -v_0 \Longrightarrow \mathrm{sen}\,\mathrm{d}\theta = -\dfrac{v_0}{2L}\,\mathrm{d}t \Longrightarrow \int\mathrm{sen}\,\mathrm{d}\theta = -\int\dfrac{v_0}{2L}\,\mathrm{d}t \Longrightarrow \cos\theta = \dfrac{v_0}{2L}\,t + C$

Para calcular el valor de la constante de integración $C$ imponemos la condición inicial

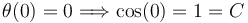
$\theta(0) = 0 \Longrightarrow \cos(0) = 1 = C$

Entonces

$\theta(t) = \arccos\left(1+\dfrac{v_0}{2L}t\right)$

Dos partículas unidas por una barra (Sep. 2018 G.I.C.)

De Laplace

Contenido

1 Enunciado

2 Solución

2.1 Vectores de posición

2.2 Velocidad y aceleración con $θ(t)$ dada

2.3 Fuerzas sobre el sistema

2.4 Energía cinética y momento cinético

2.5 Ecuación diferencial

Herramientas:

Buscar

Vistas

Herramientas

Herramientas personales

Navegación

SEARCH

TOOLBOX

LANGUAGES

Dos partículas unidas por una barra (Sep. 2018 G.I.C.)

De Laplace

Contenido

1 Enunciado

2 Solución

2.1 Vectores de posición

2.2 Velocidad y aceleración con θ(t) dada

2.3 Fuerzas sobre el sistema

2.4 Energía cinética y momento cinético

2.5 Ecuación diferencial

Herramientas:

Buscar

Vistas

Herramientas

Herramientas personales

Navegación

SEARCH

TOOLBOX

LANGUAGES

2.2 Velocidad y aceleración con $θ(t)$ dada