No Boletín - Cuestión sobre tres velocidades (Ex.Feb/14)
De Laplace
1 Enunciado
Las posiciones y velocidades instantáneas de tres puntos de un sólido rígido vienen dadas por:
| Punto | 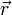 (m) (m)
| 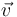 (m/s) (m/s)
|
|---|---|---|
| A | 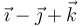
| 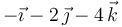
|
| B | 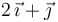
| 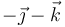
|
| C | 
| 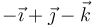
|
¿Cuál de las siguientes afirmaciones es verdadera?
(a) Estos datos son incompatibles con la rigidez
(b) La velocidad de deslizamiento (segundo invariante) vale 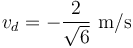
(c) La velocidad angular (primer invariante) vale 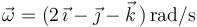
(d) El eje instantáneo de rotación pasa por el punto de coordenadas 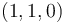
2 Equiproyectividad. Descartando (a)
La condición cinemática de rigidez consiste en la equiproyectividad del campo de velocidades:
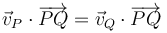
Sometiendo a examen a cada par de puntos:
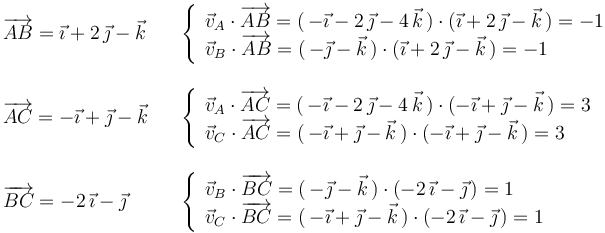
comprobamos que los datos del enunciado son compatibles con la rigidez, y por tanto la afirmación (a) es FALSA.
3 Cálculo de la velocidad angular. Descartando (c)
Podemos deducir el vector velocidad angular 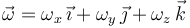 del sólido, componente a componente, exigiendo el cumplimiento de la ecuación del campo de velocidades en la relación entre las velocidades conocidas.
del sólido, componente a componente, exigiendo el cumplimiento de la ecuación del campo de velocidades en la relación entre las velocidades conocidas.
Por ejemplo, la relación entre 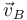 y
y 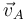 debe satisfacer la ecuación:
debe satisfacer la ecuación:
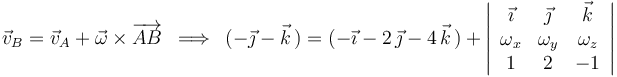
E igualando componentes homólogas:

Y relacionando de forma análoga 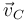 y
y 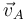 , podemos culminar la deducción de
, podemos culminar la deducción de 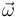 :
:
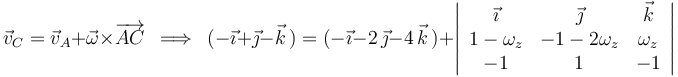
E igualando componentes homólogas:
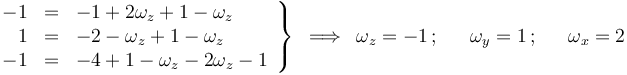
Por tanto, el vector velocidad angular (primer invariante) es:
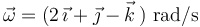
Puede observarse que el valor obtenido de 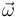 no coincide con el propuesto en la afirmación (c). En concreto, la discrepancia está en el signo de la segunda componente. Por tanto, la afirmación (c) es FALSA.
no coincide con el propuesto en la afirmación (c). En concreto, la discrepancia está en el signo de la segunda componente. Por tanto, la afirmación (c) es FALSA.





