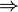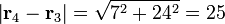Cuatro cargas en un rectángulo
De Laplace
(Diferencias entre revisiones)
(→Solución) |
(→Solución) |
||
| Línea 17: | Línea 17: | ||
La posición relativa de <math>q_4</math> respecto a las otras tres cargas y las distancias correspondientes son | La posición relativa de <math>q_4</math> respecto a las otras tres cargas y las distancias correspondientes son | ||
| + | ;De <math>q_4</math> a <math>q_1</math> | ||
<center><math>\mathbf{r}_4-\mathbf{r}_1 = 9\mathbf{u}_x-12\mathbf{u}_y</math>{{tose}}<math>\left|\mathbf{r}_4-\mathbf{r}_1\right| = \sqrt{9^2+12^2}=15</math></center> | <center><math>\mathbf{r}_4-\mathbf{r}_1 = 9\mathbf{u}_x-12\mathbf{u}_y</math>{{tose}}<math>\left|\mathbf{r}_4-\mathbf{r}_1\right| = \sqrt{9^2+12^2}=15</math></center> | ||
| + | ;De <math>q_4</math> a <math>q_2</math> | ||
| + | <center><math>\mathbf{r}_4-\mathbf{r}_2 = -16\mathbf{u}_x-12\mathbf{u}_y</math>{{tose}}<math>\left|\mathbf{r}_4-\mathbf{r}_2\right| = \sqrt{16^2+12^2}=20</math></center> | ||
| - | + | ;De <math>q_4</math> a <math>q_3</math> | |
| - | <center><math>\mathbf{r}_4-\mathbf{r} | + | <center><math>\mathbf{r}_4-\mathbf{r}_3 = -7\mathbf{u}_x-24\mathbf{u}_y</math>{{tose}}<math>\left|\mathbf{r}_4-\mathbf{r}_3\right| = \sqrt{7^2+24^2}=25</math></center> |
[[Categoría:Problemas de electrostática en el vacío]] | [[Categoría:Problemas de electrostática en el vacío]] | ||
Revisión de 17:34 8 ene 2010
1 Enunciado
Una carga puntual  se encuentra situada en el
origen de coordenadas. En
se encuentra situada en el
origen de coordenadas. En 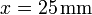 ,
,  se halla una segunda carga q2. En
se halla una segunda carga q2. En 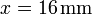 ,
, 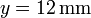 se encuentra una tercera carga q3.
se encuentra una tercera carga q3.
Calcule el valor que deben tener q2 y q3 si, ocupando las posiciones indicadas, se desea que sea nula la fuerza sobre una carga 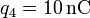 situada en
situada en 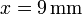 ,
,  , z = 0.
, z = 0.
2 Solución
La fuerza sobre la carga q4 es, de acuerdo con la ley de Coulomb y el principio de superposición
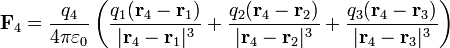
En nuestro caso, midiendo las distancias en milímetros, tenemos que
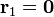
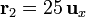

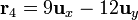
La posición relativa de q4 respecto a las otras tres cargas y las distancias correspondientes son
- De q4 a q1
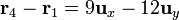
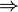
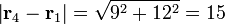
- De q4 a q2
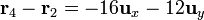
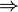
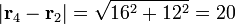
- De q4 a q3