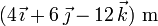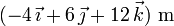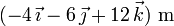1.11. Vectores con tres condiciones (Ex.Nov/11)
De Laplace
(→Solución) |
(→Solución) |
||
| Línea 24: | Línea 24: | ||
<center><math>\left|(a_x\,\vec{\imath}+a_y\,\vec{\jmath}+a_z\,\vec{k})\cdot(\vec{\imath}\wedge\vec{k})\right|=|a_y|=6\,\,\,\,\longrightarrow\,\,\,\, a_y=\pm\, 6\,\,\,\,\,\,\,\, (3)</math></center> | <center><math>\left|(a_x\,\vec{\imath}+a_y\,\vec{\jmath}+a_z\,\vec{k})\cdot(\vec{\imath}\wedge\vec{k})\right|=|a_y|=6\,\,\,\,\longrightarrow\,\,\,\, a_y=\pm\, 6\,\,\,\,\,\,\,\, (3)</math></center> | ||
| - | Sustituyendo (2) y (3) en (1), se | + | Sustituyendo (2) y (3) en (1) y resolviendo la ecuación resultante, se obtienen dos soluciones para <math>a_x\,</math>. Y a cada una de esas dos soluciones de <math>a_x\,</math> le corresponde mediante (2) una solución en <math>a_z\,</math>: |
<center><math>a_x^2+36+9a_x^2=196\,\,\,\,\longrightarrow\,\,\,\,10\,a_x^2=160\,\,\,\,\longrightarrow\,\,\,\,a_x^2=16\,\,\,\,\longrightarrow\,\,\,\,\left\{ \begin{array}{l} a_x= 4 \,\,\,\,\longrightarrow\,\,\,\, a_z=-12 \\ a_x= -4 \,\,\,\,\longrightarrow\,\,\,\, a_z=12\end{array}\right.</math></center> | <center><math>a_x^2+36+9a_x^2=196\,\,\,\,\longrightarrow\,\,\,\,10\,a_x^2=160\,\,\,\,\longrightarrow\,\,\,\,a_x^2=16\,\,\,\,\longrightarrow\,\,\,\,\left\{ \begin{array}{l} a_x= 4 \,\,\,\,\longrightarrow\,\,\,\, a_z=-12 \\ a_x= -4 \,\,\,\,\longrightarrow\,\,\,\, a_z=12\end{array}\right.</math></center> | ||
| + | |||
| + | Atendiendo a la duplicidad de signo obtenida en (3) para la componente <math>a_y\,</math>, podemos finalmente concluir que existen cuatro vectores que satisfacen las tres condiciones dadas, a saber: | ||
| + | |||
| + | <center><math>(4\,\vec{\imath}+6\,\vec{\jmath}-12\,\vec{k})\,\,\mathrm{m}</math></center> | ||
| + | |||
| + | <center><math>(4\,\vec{\imath}-6\,\vec{\jmath}-12\,\vec{k})\,\,\mathrm{m}</math></center> | ||
| + | |||
| + | <center><math>(-4\,\vec{\imath}+6\,\vec{\jmath}+12\,\vec{k})\,\,\mathrm{m}</math></center> | ||
| + | |||
| + | <center><math>(-4\,\vec{\imath}-6\,\vec{\jmath}+12\,\vec{k})\,\,\mathrm{m}</math></center> | ||
[[Categoría:Problemas de vectores libres (G.I.T.I.)]] | [[Categoría:Problemas de vectores libres (G.I.T.I.)]] | ||
Revisión de 00:39 10 nov 2011
1 Enunciado
Determine todos los vectores libres que cumplen las tres siguientes condiciones:
1) Tener una longitud de 14 m.
2) Ser ortogonal al vector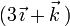 
m.
3) Formar junto a los vectores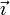 
m y
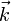m un paralelepípedo de volumen igual a 6 m3.
2 Solución
Exigiremos a un vector genérico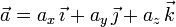 
las tres condiciones dadas. Por comodidad, prescindiremos de las unidades hasta llegar a la solución final (son todas unidades del SI).
La longitud de un vector es su módulo. Así que el cuadrado del módulo de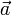 
debe ser:
La condición de ortogonalidad entre dos vectores viene dada por la nulidad de su producto escalar:
El volumen del paralelepípedo que tiene a tres vectores por aristas es igual al valor absoluto de su producto mixto:
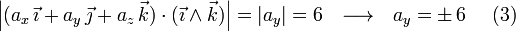
Sustituyendo (2) y (3) en (1) y resolviendo la ecuación resultante, se obtienen dos soluciones para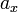 
. Y a cada una de esas dos soluciones de 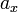le corresponde mediante (2) una solución en 
:
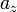Atendiendo a la duplicidad de signo obtenida en (3) para la componente 
, podemos finalmente concluir que existen cuatro vectores que satisfacen las tres condiciones dadas, a saber:
