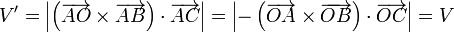1.10. Volumen de un paralelepípedo
De Laplace
| Línea 15: | Línea 15: | ||
En nuestro caso los vectores los obtenemos hallando las diferencias entre las coordenadas de cada par de puntos | En nuestro caso los vectores los obtenemos hallando las diferencias entre las coordenadas de cada par de puntos | ||
| - | <center><math>\overrightarrow{OA}= (2\vec{\imath}+2\vec{\imath}+2\vec{k})\,\mathrm{m}</math>{{qquad}}{{qquad}}<math>\overrightarrow{OB}= (\vec{\imath}+6\vec{\imath}+6\vec{k})\,\mathrm{m}</math>{{qquad}}{{qquad}}<math>\overrightarrow{OC}= (\vec{\imath}-3\vec{\imath}-\vec{k})\,\mathrm{m}</math></center> | + | <center><math>\overrightarrow{OA}= (2\vec{\imath}+2\vec{\imath}+2\vec{k})\,\mathrm{m}</math>{{qquad}}{{qquad}}<math>\overrightarrow{OB}= (\vec{\imath}+6\vec{\imath}+6\vec{k})\,\mathrm{m}</math>{{qquad}}{{qquad}}<math>\overrightarrow{OC}= (\vec{\imath}-3\vec{\imath}-1\vec{k})\,\mathrm{m}</math></center> |
de forma que el producto mixto lo da el determinante | de forma que el producto mixto lo da el determinante | ||
| Línea 28: | Línea 28: | ||
[[Archivo:volumen-paralelepipedo-02.png|right]] | [[Archivo:volumen-paralelepipedo-02.png|right]] | ||
| + | |||
| + | Para el segundo paralelepípedo calculamos los nuevos vectores de posición relativos | ||
| + | |||
| + | <center><math>\overrightarrow{AO}= (-2\vec{\imath}-2\vec{\imath}-2\vec{k})\,\mathrm{m}</math>{{qquad}}{{qquad}}<math>\overrightarrow{AB}= (-\vec{\imath}+4\vec{\imath}+4\vec{k})\,\mathrm{m}</math>{{qquad}}{{qquad}}<math>\overrightarrow{AC}= (-\vec{\imath}-5\vec{\imath}-3\vec{k})\,\mathrm{m}</math></center> | ||
| + | |||
| + | El nuevo producto mixto es | ||
| + | |||
| + | <center><math>\overrightarrow{AO}\cdot\left(\overrightarrow{AB}\times\overrightarrow{AC}\right) = \left|\begin{matrix}-2 & -2 & -2 \\ -1 & 4 & 4\\ -1 & -5 & -3\end{matrix}\right|\,\mathrm{m}^3=-20\,\mathrm{m}^3</math></center> | ||
| + | |||
| + | Puesto que el volumen debe ser positivo obtenemos | ||
| + | |||
| + | <center><math>V' = 20\,\mathrm{m}^3</math></center> | ||
| + | |||
| + | Este es el mismo resultado que antes, pese a que geométricamente estemos hablando de un paralelepípedo diferente. Puede demostrarse en general: un paralelepípedo definido por cuatro puntos a partir de tres vectores concurrentes que unen uno de los puntos con los otros tres posee el mismo volumen sea cual sea el punto de los cuatro que tomemos como origen de los vectores. | ||
| + | |||
| + | Para este caso la demostración parte de | ||
| + | |||
| + | <center><math>V' = \left|\left(\overrightarrow{AO}\times\overrightarrow{AB}\right)\cdot\overrightarrow{AC}\right|</math></center> | ||
| + | |||
| + | aplicando que | ||
| + | |||
| + | <center><math>\overrightarrow{AO}=-\overrightarrow{OA}</math>{{qquad}}{{qquad}}<math>\overrightarrow{AB}=\overrightarrow{OB}-\overrightarrow{OA}</math></center> | ||
| + | |||
| + | nos queda, para el primer producto vectorial | ||
| + | |||
| + | <center><math>\overrightarrow{AO}\times\overrightarrow{AB} = -\overrightarrow{OA}\times\left(\overrightarrow{OB}-\overrightarrow{OA}\right) = -\overrightarrow{OA}\times\overrightarrow{OB}</math></center> | ||
| + | |||
| + | ya que el producto vectorial de un vector por si mismo es nulo. Aplicando ahora que | ||
| + | |||
| + | <center><math>\overrightarrow{AC}=\overrightarrow{OC}-\overrightarrow{OA}</math></center> | ||
| + | |||
| + | y que el producto vectorial de <math>\overrightarrow{OA}</math> por cualquier cosa es ortogonal a <math>\overrightarrow{OA}</math> llegamos a | ||
| + | |||
| + | <center><math>\left(\overrightarrow{OA}\times\overrightarrow{OB})\cdot\left(\overrightarrow{OC}-\overrightarrow{OA}\right) = \left(\overrightarrow{OA}\times\overrightarrow{OB})\cdot\overrightarrow{OC}</math></center> | ||
| + | |||
| + | Concluimos entonces que | ||
| + | |||
| + | <center><math>V' = \left|\left(\overrightarrow{AO}\times\overrightarrow{AB}\right)\cdot\overrightarrow{AC}\right|=\left|-\left(\overrightarrow{OA}\times\overrightarrow{OB}\right)\cdot\overrightarrow{OC}\right|=V</math></center> | ||
| + | |||
==Volumen del tetraedro== | ==Volumen del tetraedro== | ||
[[Categoría:Problemas de vectores libres (G.I.T.I.)]] | [[Categoría:Problemas de vectores libres (G.I.T.I.)]] | ||
Revisión de 10:40 25 sep 2010
Contenido |
1 Enunciado
Sean los puntos de coordenadas (en el SI) O(1,0,2), A(3,2,4), B(2,6,8) y C(2, − 3,1). Determine el volumen del paralelepípedo definido por los vectores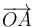 
, 
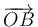y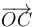 
.
Halle del mismo modo el volumen del paralelepípedo definido por los vectores 
, 
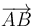y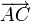 
.
Calcule igualmente el volumen del tetraedro irregular definido por estos cuatro puntos.
2 Primer volumen
El volumen de un paralelepípedo se calcula como el producto mixto (sin signo) de los tres vectores que definen el paralelepíedo.
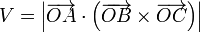
En nuestro caso los vectores los obtenemos hallando las diferencias entre las coordenadas de cada par de puntos
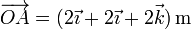
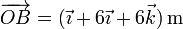
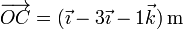
de forma que el producto mixto lo da el determinante
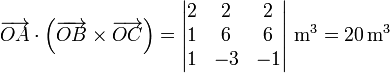
Al ser positivo, este es el volumen del paralelepípedo.
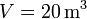
3 Segundo volumen
Para el segundo paralelepípedo calculamos los nuevos vectores de posición relativos
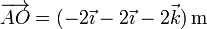
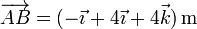
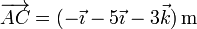
El nuevo producto mixto es
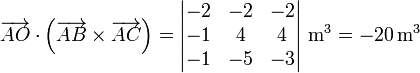
Puesto que el volumen debe ser positivo obtenemos
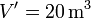
Este es el mismo resultado que antes, pese a que geométricamente estemos hablando de un paralelepípedo diferente. Puede demostrarse en general: un paralelepípedo definido por cuatro puntos a partir de tres vectores concurrentes que unen uno de los puntos con los otros tres posee el mismo volumen sea cual sea el punto de los cuatro que tomemos como origen de los vectores.
Para este caso la demostración parte de
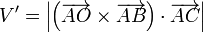
aplicando que
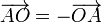
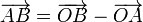
nos queda, para el primer producto vectorial
ya que el producto vectorial de un vector por si mismo es nulo. Aplicando ahora que
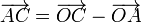
y que el producto vectorial de 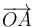por cualquier cosa es ortogonal a 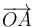llegamos a
Concluimos entonces que
