No Boletín - Afirmación falsa (Ex.Nov/16)
De Laplace
(Diferencias entre revisiones)
(→Enunciado) |
|||
| Línea 13: | Línea 13: | ||
==Solución== | ==Solución== | ||
| + | Las coordenadas de un punto en un sistema de ejes cartesianos son las componentes de su vector de posición en la base ortonormal asociada, es decir: | ||
| + | <center><math> | ||
| + | A(2,4,0)\,\,\,\longrightarrow\,\,\,\overrightarrow{OA}=2\,\vec{\imath}+4\,\vec{\jmath}\,;\,\,\,\,\,\,\,\,\,\, | ||
| + | B(0,2,2)\,\,\,\longrightarrow\,\,\,\overrightarrow{OB}=2\,\vec{\jmath}+2\,\vec{k}\,;\,\,\,\,\,\,\,\,\,\, | ||
| + | C(-1,0,\mathrm{p})\,\,\,\longrightarrow\,\,\,\overrightarrow{OC}=-\vec{\imath}+\mathrm{p}\,\vec{k} | ||
| + | </math></center> | ||
| + | Y, por otra parte: | ||
| + | <center><math> | ||
| + | \overrightarrow{AB}=\overrightarrow{OB}-\overrightarrow{OA}=(-2\,\vec{\imath}-2\,\vec{\jmath}+2\,\vec{k}\,;\,\,\,\,\,\,\,\,\,\,\overrightarrow{BC}=\overrightarrow{OC}-\overrightarrow{OB}=-\vec{\imath}-2\,\vec{\jmath}+(\mathrm{p}-2)\,\vec{k} | ||
| + | </math></center> | ||
| + | Exigiendo la condición de ortogonalidad (producto escalar nulo) a los vectores <math>\overrightarrow{OB}\,</math> y <math>\overrightarrow{BC}\,</math>: | ||
| + | <center><math> | ||
| + | \overrightarrow{OB}\cdot\overrightarrow{BC}=0\,\,\,\,\,\Longrightarrow\,\,\,\,\,[2\,\vec{\jmath}+2\,\vec{k}]\cdot[-\vec{\imath}-2\,\vec{\jmath}+(\mathrm{p}-2)\,\vec{k}]=0\,\,\,\,\,\Longrightarrow\,\,\,\,\,2\mathrm{p}-8=0\,\,\,\,\,\Longrightarrow\,\,\,\,\,\mathrm{p}=4 | ||
| + | </math></center> | ||
| + | Por tanto, la afirmación (2) es correcta. | ||
| + | Exigiendo la condición de paralelismo (producto vectorial nulo) a los vectores <math>\overrightarrow{OA}\,</math> y <math>\overrightarrow{BC}\,</math>: | ||
| + | <center><math> | ||
| + | \overrightarrow{OA}\times\overrightarrow{BC}=\vec{0}\,\,\,\,\,\Longrightarrow\,\,\,\,\,\left|\begin{array}{ccc} \vec{\imath} & \vec{\jmath} & \vec{k} \\ 2 & 4 & 0 \\ -1 & -2 & (\mathrm{p}-2) \end{array}\right|=\vec{0}\,\,\,\,\,\Longrightarrow\,\,\,\,\,(4\mathrm{p}-8)\,\vec{\imath}+(-2\mathrm{p}+4)\,\vec{\jmath}=\vec{0}\,\,\,\,\,\Longrightarrow\,\,\,\,\,\mathrm{p}=2 | ||
| + | </math></center> | ||
| + | Por tanto, la afirmación (4) es correcta. | ||
| + | Exigiendo la condición de no coplanariedad (producto mixto no nulo) a los vectores <math>\overrightarrow{OA}\,</math>, <math>\overrightarrow{OB}\,</math> y <math>\overrightarrow{OC}\,</math>, se garantiza que dicha terna constituya una base: | ||
| + | <center><math> | ||
| + | \overrightarrow{OA}\cdot(\overrightarrow{OB}\times\overrightarrow{OC})\neq 0\,\,\,\,\,\Longrightarrow\,\,\,\,\,\left|\begin{array}{ccc} 2 & 4 & 0 \\ 0 & 2 & 2 \\ -1 & 0 & \mathrm{p} \end{array}\right|\neq 0\,\,\,\,\,\Longrightarrow\,\,\,\,\,4\mathrm{p}-8\neq 0\,\,\,\,\,\Longrightarrow\,\,\,\,\,\mathrm{p}\neq 2 | ||
| + | </math></center> | ||
| + | Por tanto, la afirmación (1) es correcta. | ||
| + | Por último, exigiendo la condición de coplanariedad (producto mixto nulo) a los vectores <math>\overrightarrow{OA}\,</math>, <math>\overrightarrow{AB}\,</math> y <math>\overrightarrow{BC}\,</math>: | ||
| + | <center><math> | ||
| + | \overrightarrow{OA}\cdot(\overrightarrow{AB}\times\overrightarrow{BC})= 0\,\,\,\,\,\Longrightarrow\,\,\,\,\,\left|\begin{array}{ccc} 2 & 4 & 0 \\ -2 & -2 & 2 \\ -1 & -2 & (\mathrm{p}-2) \end{array}\right|=-12\mathrm{p}+24= 0\,\,\,\,\,\Longrightarrow\,\,\,\,\,\mathrm{p}=2 | ||
| + | </math></center> | ||
| + | Por tanto, la afirmación (3) es la que es FALSA. | ||
[[Categoría:Problemas de vectores libres (G.I.T.I.)]] | [[Categoría:Problemas de vectores libres (G.I.T.I.)]] | ||
Revisión de 20:59 1 mar 2017
1 Enunciado
En un triedro cartesiano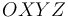 
se consideran los siguientes puntos: 
, 
, 
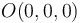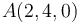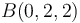y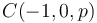 
.
¿Cuál de las siguientes afirmaciones es falsa?
- (1)
, 
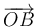y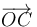 
constituyen una base si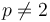 
- (2) 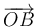y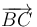
son ortogonales si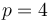 
- (3) ,
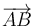y 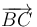son coplanarios si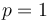 
- (4) y 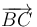son paralelos si
2 Solución
Las coordenadas de un punto en un sistema de ejes cartesianos son las componentes de su vector de posición en la base ortonormal asociada, es decir:
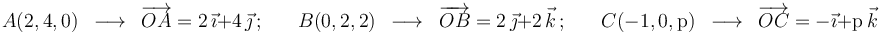
Y, por otra parte:
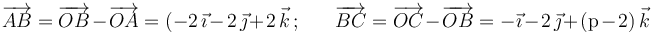
Exigiendo la condición de ortogonalidad (producto escalar nulo) a los vectores 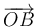y :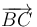
![\overrightarrow{OB}\cdot\overrightarrow{BC}=0\,\,\,\,\,\Longrightarrow\,\,\,\,\,[2\,\vec{\jmath}+2\,\vec{k}]\cdot[-\vec{\imath}-2\,\vec{\jmath}+(\mathrm{p}-2)\,\vec{k}]=0\,\,\,\,\,\Longrightarrow\,\,\,\,\,2\mathrm{p}-8=0\,\,\,\,\,\Longrightarrow\,\,\,\,\,\mathrm{p}=4](/wiki/images/math/d/6/8/d68c956de405e105cc497637b07fc07a.png)
Por tanto, la afirmación (2) es correcta.
Exigiendo la condición de paralelismo (producto vectorial nulo) a los vectores y :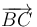
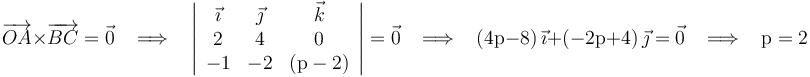
Por tanto, la afirmación (4) es correcta.
Exigiendo la condición de no coplanariedad (producto mixto no nulo) a los vectores , 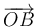y 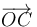, se garantiza que dicha terna constituya una base:
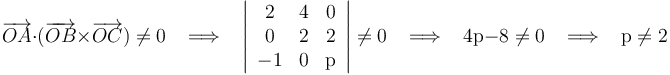
Por tanto, la afirmación (1) es correcta.
Por último, exigiendo la condición de coplanariedad (producto mixto nulo) a los vectores , 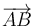y :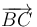
Por tanto, la afirmación (3) es la que es FALSA.
