Cálculo numérico de la derivada del seno

(Diferencias entre revisiones)

Revisión de 21:56 5 oct 2011

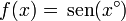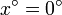Se trata de calcular la derivada de $f(x)=\,\mathrm{sen}(x^\circ)$ para $x^\circ=0^\circ$ .

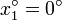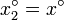Exprese el cociente $Δ f / Δ x$ , cuando $x_1^\circ=0^\circ$ y $x_2^\circ=x^\circ$ .
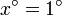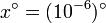Calcule numéricamente el cociente anterior para $x^\circ=1^\circ$ , $x^\circ=0.1^\circ$ , $x^\circ=0.01^\circ$ ,… hasta $x^\circ=(10^{-6})^\circ$ . ¿A cuanto tiende el límite?
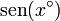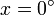Multiplique los resultados anteriores por 180. A la vista de los resultados, ¿cuanto vale la derivada de $\mathrm{sen}(x^\circ)$ en $x=0^\circ$ ?

La derivada de una función equivale al límite del cociente entre incrementos cuando estos tienden a cero

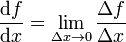
$\frac{\mathrm{d}f}{\mathrm{d}x} = \lim_{\Delta x\to 0} \frac{\Delta f}{\Delta x}$

En nuestro caso, consideramos un incremento entre 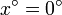$x^\circ = 0^\circ$ y un cierto valor del ángulo

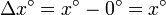
$\Delta x^\circ = x^\circ - 0^\circ = x^\circ$

mientras que el incremento en la función es

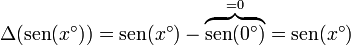
$\Delta(\mathrm{sen}(x^\circ))=\mathrm{sen}(x^\circ)-\overbrace{\mathrm{sen}(0^\circ)}^{=0}= \mathrm{sen}(x^\circ)$

Por tanto, el cociente entre incrementos se reduce a

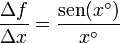
$\frac{\Delta f}{\Delta x}=\frac{\mathrm{sen}(x^\circ)}{x^\circ}$

Calculamos entonces los valores del cociente incremental para valores cada vez más pequeños del argumento

@@ Línea 22: / Línea 22: @@
 <center><math>\frac{\Delta f}{\Delta x}=\frac{\mathrm{sen}(x^\circ)}{x^\circ}</math></center>
+==Aproximación numérica==
+Calculamos entonces los valores del cociente incremental para valores cada vez más pequeños del argumento
+{| class="bordeado"
+|-
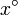
+! <math>x^\circ</math>
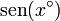
+! <math>\mathrm{sen}(x^\circ)</math>
+! <math>\mathrm{sen}(x^\circ)/\Delta x</math>
+|-
+| 1
+| 0.017452406437283512819
+| 0.017452406437283512819
+|-
+| 0.1
+| 0.001745328365898308836
+| 0.017453283658983088358
+|-
+| 0.01
+| 0.001745329243133368033
+| 0.017453292431333680334
+|-
+| 0.001
+| 0.000174532925190571996
+| 0.017453292519057199614
+|-
+| 0.0001
+| 0.000017453292519934435
+| 0.017453292519934434808
+|-
+| 0.00001
+| 0.000001745329251994321
+| 0.017453292519943207160
+|-
+| 0.000001
+| 0.000000174532925199433
+| 0.017453292519943294883
+|}
 [[Categoría:Problemas de herramientas matemáticas (GIE)]]