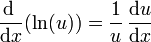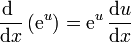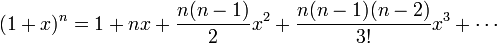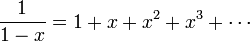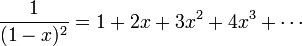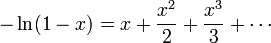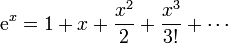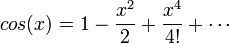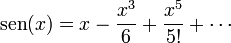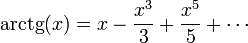Entrar Página Discusión Historial Go to the site toolbox

Tabla de derivadas y primitivas

De Laplace

(Diferencias entre revisiones)
(Página creada con '==Derivadas== {| class="bordeado" |- ! <math>f(x)</math> ! <math>\mathrm{d}f/\mathrm{d}x</math> ! <math>f(x)</math> ! <math>\mathrm{d}f/\mathrm{d}x</math> |- | <math>C</math> | …')
(→Tabla de primitivas)
 
(19 ediciones intermedias no se muestran.)
Línea 1: Línea 1:
-
==Derivadas==
+
==Reglas de derivación==
 +
;Suma de funciones
 +
:<math>\frac{\mathrm{d}\ }{\mathrm{d}x}(u+v) = \frac{\mathrm{d}u}{\mathrm{d}x} + \frac{\mathrm{d}v}{\mathrm{d}x}</math>
 +
 
 +
;Producto de funciones
 +
:<math>\frac{\mathrm{d}\ }{\mathrm{d}x}(uv) = \left(\frac{\mathrm{d}u}{\mathrm{d}x}\right)v + u\left(\frac{\mathrm{d}v}{\mathrm{d}x}\right)</math>
 +
 
 +
Caso particular <math>u = C = \mathrm{cte}</math>
 +
 
 +
:<math>\frac{\mathrm{d}\ }{\mathrm{d}x}(Cv) = C\left(\frac{\mathrm{d}v}{\mathrm{d}x}\right)</math>
 +
 
 +
;Regla de la cadena
 +
 
 +
:<math>\frac{\mathrm{d}v}{\mathrm{d}x}=\frac{\mathrm{d}v}{\mathrm{d}u}\,\frac{\mathrm{d}u}{\mathrm{d}x}</math>
 +
 
 +
Caso particular de derivada logarítmica
 +
:<math>\frac{\mathrm{d}\ }{\mathrm{d}x}(\ln(u)) = \frac{1}{u}\,\frac{\mathrm{d}u}{\mathrm{d}x}</math>
 +
 
 +
Caso particular de exponencial de una función
 +
:<math>\frac{\mathrm{d}\ }{\mathrm{d}x}\left(\mathrm{e}^u\right) = \mathrm{e}^u\,\frac{\mathrm{d}u}{\mathrm{d}x}</math>
 +
 
 +
==Tabla de derivadas==
{| class="bordeado"
{| class="bordeado"
|-
|-
Línea 7: Línea 28:
! <math>\mathrm{d}f/\mathrm{d}x</math>
! <math>\mathrm{d}f/\mathrm{d}x</math>
|-
|-
-
| <math>C</math>
+
| <math>C\,</math>
-
| <math>0</math>
+
| <math>0\,</math>
-
|  
+
| <math>\ln(x)\,</math>
-
|  
+
| <math>\frac{1}{x}</math>
|-
|-
-
| <math>x</math>
+
| <math>x\,</math>
-
| <math>1</math>
+
| <math>1\,</math>
-
|  
+
| <math>\mathrm{sen}(x)\,</math>
-
|  
+
| <math>\cos(x)\,</math>
|-
|-
-
| <math>x^2</math>
+
| <math>x^2\,</math>
-
| <math>2x</math>
+
| <math>2x\,</math>
-
|  
+
| <math>\cos(x)\,</math>
-
|  
+
| <math>-\mathrm{sen}(x)\,</math>
|-
|-
| <math>\frac{1}{x}</math>
| <math>\frac{1}{x}</math>
| <math>-\frac{1}{x^2}</math>
| <math>-\frac{1}{x^2}</math>
-
|  
+
| <math>\mathrm{tg}(x)\,</math>
-
|  
+
| <math>\frac{1}{\cos^2(x)}</math>
|-
|-
-
| <math>x^n</math>
+
| <math>x^n\,</math>
-
| <math>nx^{n-1}</math>
+
| <math>nx^{n-1}\,</math>
-
|  
+
| <math>\mathrm{arcsen}(x)\,</math>
-
|  
+
| <math>\displaystyle\frac{1}{\sqrt{1-x^2}}</math>
|-
|-
-
| <math>\ln(x)</math>
+
| <math>\mathrm{e}^x\,</math>
-
| <math>\frac{1}{x}</math>
+
| <math>\mathrm{e}^x\,</math>
-
|
+
| <math>\mathrm{arccos}(x)\,</math>
-
|
+
| <math>\displaystyle -\frac{1}{\sqrt{1-x^2}}</math>
|-
|-
-
| <math>\mathrm{e}^x</math>
+
| <math>a^x\,</math>
-
| <math>\mathrm{e}^x</math>
+
| <math>\ln(a)\,a^x</math>
-
|  
+
| <math>\mathrm{arctg}(x)\,</math>
-
|  
+
| <math>\displaystyle\frac{1}{1+x^2}</math>
 +
|}
 +
 
 +
==Tabla de primitivas==
 +
{| class="bordeado"
|-
|-
-
| <math>\cos(x)</math>
+
! <math>f(x)</math>
-
| <math>-\mathrm{sen}(x)</math>
+
! <math>\int f\,\mathrm{d}x</math>
-
|
+
! <math>f(x)</math>
-
|
+
! <math>\int f\,\mathrm{d}x</math>
|-
|-
-
| <math>\mathrm{sen}(x)</math>
+
| <math>k\,</math>
-
| <math>\cos(x)</math>
+
| <math>kx+C\,</math>
-
|
+
| <math>\mathrm{sen}(x)\,</math>
-
|
+
| <math>-\cos(x)+C\,</math>
|-
|-
-
| <math>\mathrm{tg}(x)</math>
+
| <math>x\,</math>
-
| <math>\frac{1}{\cos^2(x)}</math>
+
| <math>\frac{x^2}{2}+C\,</math>
-
|  
+
| <math>\cos(x)\,</math>
-
|
+
| <math>\mathrm{sen}(x)+C\,</math>
-
||}
+
|-
 +
| <math>\frac{1}{x}\,</math>
 +
| <math>\ln|x|+C\,</math>
 +
| <math>\mathrm{tg}(x)\,</math>
 +
| <math>-\ln|\cos(x)|+C\,</math>
 +
|-
 +
| <math>x^n \quad (n\neq -1)</math>
 +
| <math>\frac{x^{n+1}}{n+1}+C</math>
 +
| <math>\frac{1}{\mathrm{sen}(x)}</math>
 +
| <math>\ln\left|\mathrm{tg}\left(\frac{x}{2}\right)\right|+C</math>
 +
|-
 +
| <math>\mathrm{e}^x\,</math>
 +
| <math>\mathrm{e}^x+C\,</math>
 +
| <math>\frac{1}{1+x^2}</math>
 +
| <math>\mathrm{arctg}(x)+C\,</math>
 +
|-
 +
| <math>\mathrm{e}^{\lambda x}\,</math>
 +
| <math>\frac{1}{\lambda}\mathrm{e}^{\lambda x}+C\,</math>
 +
| <math>\displaystyle\frac{1}{\sqrt{1-x^2}}</math>
 +
| <math>\mathrm{arcsen}(x)+C\,</math>
 +
|}
 +
 
 +
Más primitivas en la [http://en.wikipedia.org/wiki/Lists_of_integrals Wikipedia]
 +
 
 +
==Series de Taylor==
 +
 
 +
:<math>(1+x)^n = 1 + n x + \frac{n(n-1)}{2}x^2+\frac{n(n-1)(n-2)}{3!}x^3+ \cdots</math>
 +
 
 +
:<math>\frac{1}{1-x}=1+x+x^2+x^3+\cdots </math>
 +
 
 +
:<math>\frac{1}{(1-x)^2}=1+2x+3x^2+4x^3+\cdots </math>
 +
 
 +
:<math>-\ln(1-x)=x+\frac{x^2}{2}+\frac{x^3}{3}+\cdots </math>
 +
 
 +
:<math>\mathrm{e}^x=1+x+\frac{x^2}{2}+\frac{x^3}{3!}+\cdots </math>
 +
 
 +
:<math>cos(x)=1-\frac{x^2}{2}+\frac{x^4}{4!}+\cdots </math>
 +
 
 +
:<math>\mathrm{sen}(x)=x-\frac{x^3}{6}+\frac{x^5}{5!}+\cdots </math>
 +
 
 +
:<math>\mathrm{arctg}(x)=x-\frac{x^3}{3}+\frac{x^5}{5}+\cdots </math>
 +
 
 +
[[Categoría:Herramientas matemáticas (GIE)]]

última version al 17:31 11 oct 2011

Contenido

[ocultar]

1 Reglas de derivación

Suma de funciones
\frac{\mathrm{d}\ }{\mathrm{d}x}(u+v) = \frac{\mathrm{d}u}{\mathrm{d}x} + \frac{\mathrm{d}v}{\mathrm{d}x}
Producto de funciones
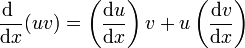
\frac{\mathrm{d}\ }{\mathrm{d}x}(uv) = \left(\frac{\mathrm{d}u}{\mathrm{d}x}\right)v + u\left(\frac{\mathrm{d}v}{\mathrm{d}x}\right)

Caso particular u = C = cte

\frac{\mathrm{d}\ }{\mathrm{d}x}(Cv) = C\left(\frac{\mathrm{d}v}{\mathrm{d}x}\right)
Regla de la cadena
\frac{\mathrm{d}v}{\mathrm{d}x}=\frac{\mathrm{d}v}{\mathrm{d}u}\,\frac{\mathrm{d}u}{\mathrm{d}x}

Caso particular de derivada logarítmica

\frac{\mathrm{d}\ }{\mathrm{d}x}(\ln(u)) = \frac{1}{u}\,\frac{\mathrm{d}u}{\mathrm{d}x}

Caso particular de exponencial de una función

\frac{\mathrm{d}\ }{\mathrm{d}x}\left(\mathrm{e}^u\right) = \mathrm{e}^u\,\frac{\mathrm{d}u}{\mathrm{d}x}

2 Tabla de derivadas

f(x) df / dx f(x) df / dx
C\, 0\, \ln(x)\, \frac{1}{x}
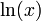
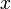
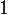
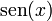
x\, 1\, \mathrm{sen}(x)\, \cos(x)\,
x^2\, 2x\, \cos(x)\, -\mathrm{sen}(x)\,
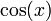
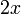
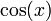
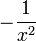
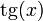
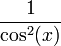
\frac{1}{x} -\frac{1}{x^2} \mathrm{tg}(x)\, \frac{1}{\cos^2(x)}
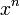
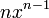
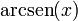
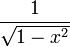
x^n\, nx^{n-1}\, \mathrm{arcsen}(x)\, \displaystyle\frac{1}{\sqrt{1-x^2}}
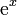
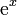
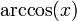
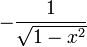
\mathrm{e}^x\, \mathrm{e}^x\, \mathrm{arccos}(x)\, \displaystyle -\frac{1}{\sqrt{1-x^2}}
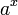
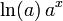
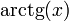
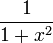
a^x\, \ln(a)\,a^x \mathrm{arctg}(x)\, \displaystyle\frac{1}{1+x^2}

3 Tabla de primitivas

f(x) 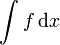\int f\,\mathrm{d}x f(x) 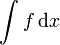\int f\,\mathrm{d}x
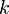
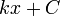
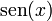
k\, kx+C\, \mathrm{sen}(x)\, -\cos(x)+C\,
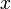
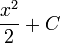
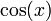
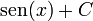
x\, \frac{x^2}{2}+C\, \cos(x)\, \mathrm{sen}(x)+C\,
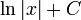
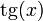
\frac{1}{x}\, \ln|x|+C\, \mathrm{tg}(x)\, -\ln|\cos(x)|+C\,
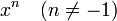
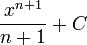
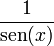
x^n \quad (n\neq -1) \frac{x^{n+1}}{n+1}+C \frac{1}{\mathrm{sen}(x)} \ln\left|\mathrm{tg}\left(\frac{x}{2}\right)\right|+C
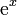
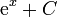
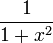
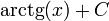
\mathrm{e}^x\, \mathrm{e}^x+C\, \frac{1}{1+x^2} \mathrm{arctg}(x)+C\,
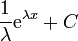
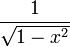
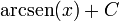
\mathrm{e}^{\lambda x}\, \frac{1}{\lambda}\mathrm{e}^{\lambda x}+C\, \displaystyle\frac{1}{\sqrt{1-x^2}} \mathrm{arcsen}(x)+C\,

Más primitivas en la Wikipedia

4 Series de Taylor

(1+x)^n = 1 + n x + \frac{n(n-1)}{2}x^2+\frac{n(n-1)(n-2)}{3!}x^3+ \cdots
\frac{1}{1-x}=1+x+x^2+x^3+\cdots
\frac{1}{(1-x)^2}=1+2x+3x^2+4x^3+\cdots
-\ln(1-x)=x+\frac{x^2}{2}+\frac{x^3}{3}+\cdots
\mathrm{e}^x=1+x+\frac{x^2}{2}+\frac{x^3}{3!}+\cdots
cos(x)=1-\frac{x^2}{2}+\frac{x^4}{4!}+\cdots
\mathrm{sen}(x)=x-\frac{x^3}{6}+\frac{x^5}{5!}+\cdots
\mathrm{arctg}(x)=x-\frac{x^3}{3}+\frac{x^5}{5}+\cdots
Obtenido de "http://tesla.us.es/wiki/index.php/Tabla_de_derivadas_y_primitivas"

Herramientas:

Herramientas personales
TOOLBOX
LANGUAGES
licencia de Creative Commons
 Esta página fue modificada por última vez el 17:31, 11 oct 2011. - Esta página ha sido visitada 29.623 veces. - Aviso legal - Acerca de Laplace