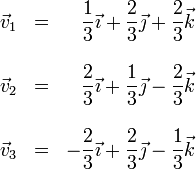1.12. Ejemplo de construcción de una base

De Laplace

(Diferencias entre revisiones)

Revisión de 16:03 7 sep 2010

Contenido

1 Enunciado
2 Primer vector
3 Segundo vector
4 Tercer vector

1 Enunciado

Dados los vectores

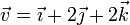
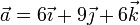
$\vec{v}=\vec{\imath}+2\vec{\jmath}+2\vec{k}$ $\vec{a}=6\vec{\imath}+9\vec{\jmath}+6\vec{k}$

Construya una base ortonormal dextrógira, tal que

El primer vector vaya en la dirección de $\vec{v}$
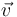
El segundo esté contenido en el plano definido por 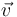$\vec{v}$ y $\vec{a}$
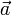
El tercero sea perpendicular a los dos anteriores, y orientado según la regla de la mano derecha.

2 Primer vector

Obtenemos el primer vector normalizando el vector 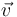$\vec{v}$ , esto es, hallando el unitario en su dirección y sentido, lo que se consigue dividiendo este vector por su módulo

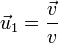
$\vec{u}_1=\frac{\vec{v}}{v}$

Hallamos el módulo de 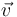$\vec{v}$

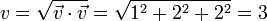
$v = \sqrt{\vec{v}\cdot\vec{v}}=\sqrt{1^2+2^2+2^2}=3$

por lo que

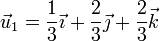
$\vec{u}_1 = \frac{1}{3}\vec{\imath}+\frac{2}{3}\vec{\jmath}+\frac{2}{3}\vec{k}$

3 Segundo vector

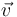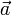El segundo vector debe estar en el plano definido por $\vec{v}$ y $\vec{a}$ , por lo que debe ser una combinación lineal de ambos

$\vec{u}_2 = \lambda\vec{v}+\mu\vec{a}$

además debe ser ortogonal a 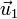$\vec{u}_1$ (y por tanto, a $\vec{v}$ )

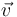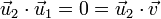
$\vec{u}_2\cdot\vec{u}_1 = 0 = \vec{u}_2\cdot\vec{v}$

y debe ser unitario

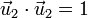
$\vec{u}_2\cdot\vec{u}_2=1$

El procedimiento sistemático consiste en hallar la componente de 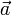$\vec{a}$ normal a 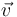$\vec{v}$ y posteriormente normalizar el resultado.

La proyección normal la calculamos con ayuda del doble producto vectorial

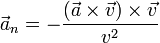
$\vec{a}_n = -\frac{(\vec{a}\times\vec{v})\times\vec{v}}{v^2}$

Calculamos el primer producto vectorial

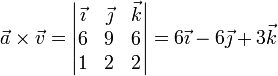
$\vec{a}\times\vec{v}=\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ 6 & 9 & 6\\ 1 & 2 & 2\end{matrix}\right|=6\vec{\imath}-6\vec{\jmath}+3\vec{k}$

Hallamos el segundo

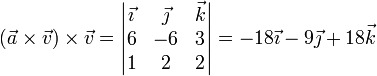
$(\vec{a}\times\vec{v})\times \vec{v}=\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ 6 & -6 & 3 \\ 1 & 2 & 2 \end{matrix}\right|=-18\vec{\imath}-9\vec{\jmath}+18\vec{k}$

Dividiendo por el módulo de 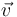$\vec{v}$ al cuadrado y cambiando el signo obtenemos la componente normal

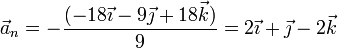
$\vec{a}_n = -\frac{(-18\vec{\imath}-9\vec{\jmath}+18\vec{k})}{9}=2\vec{\imath}+\vec{\jmath}-2\vec{k}$

Normalizando esta cantidad obtenemos el segundo vector de la base

$\vec{u}_2 = \frac{\vec{a}_n}{a_n}=\frac{2}{3}\vec{\imath}+\frac{1}{3}\vec{\jmath}-\frac{2}{3}\vec{k}$

4 Tercer vector

El tercer vector lo obtenemos como el producto vectorial de los dos primeros

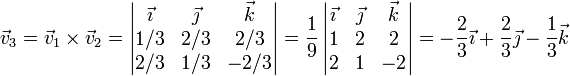
$\vec{v}_3=\vec{v}_1\times\vec{v}_2=\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ 1/3 & 2/3 & 2/3 \\ 2/3 & 1/3 & -2/3\end{matrix}\right|=\frac{1}{9}\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ 1 & 2 & 2 \\ 2 & 1 & -2\end{matrix}\right| = -\frac{2}{3}\vec{\imath}+\frac{2}{3}\vec{\jmath}-\frac{1}{3}\vec{k}$

Por tanto, la base ortonormal dextrógira está formada por los vectores

$\begin{array}{lcr} \vec{v}_1 & = & \displaystyle\frac{1}{3}\vec{\imath}+\displaystyle\frac{2}{3}\vec{\jmath}+\displaystyle\frac{2}{3}\vec{k}\\&& \\ \vec{v}_2 & = & \displaystyle\frac{2}{3}\vec{\imath}+\displaystyle\frac{1}{3}\vec{\jmath}-\displaystyle\frac{2}{3}\vec{k}\\&& \\ \vec{v}_3 & = & -\displaystyle\frac{2}{3}\vec{\imath}+\displaystyle\frac{2}{3}\vec{\jmath}-\displaystyle\frac{1}{3}\vec{k} \end{array}$

@@ Línea 50: / Línea 50: @@
 <center><math>(\vec{a}\times\vec{v})\times \vec{v}=\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ 6 & -6 & 3 \\ 1 & 2 & 2  \end{matrix}\right|=-18\vec{\imath}-9\vec{\jmath}+18\vec{k}</math></center>
-Dividiendo por el módulo de \vec{v} al cuadrado y cambiando el signo obtenemos la componente normal
+Dividiendo por el módulo de <math>\vec{v}</math> al cuadrado y cambiando el signo obtenemos la componente normal
 <center><math>\vec{a}_n = -\frac{(-18\vec{\imath}-9\vec{\jmath}+18\vec{k})}{9}=2\vec{\imath}+\vec{\jmath}-2\vec{k}</math></center>

1.12. Ejemplo de construcción de una base

De Laplace

Revisión de 16:03 7 sep 2010

Contenido

1 Enunciado

2 Primer vector

3 Segundo vector

4 Tercer vector

Herramientas:

Buscar

Vistas

Herramientas

Herramientas personales

Navegación

SEARCH

TOOLBOX

LANGUAGES