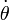Espiral logarítmica

De Laplace

(Diferencias entre revisiones)

Revisión de 16:08 25 jun 2010

Contenido

1 Enunciado
2 Ley horaria
3 Tiempo en llegar al origen
4 Aceleración
5 Centros de curvatura

1 Enunciado

Una partícula recorre la espiral logarítmica de ecuación

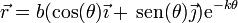
$\vec{r} = b (\cos(\theta)\vec{\imath}+\,\mathrm{sen}(\theta)\vec{\jmath})\mathrm{e}^{-k\theta}$

donde $b$ y $k$ son constantes. El movimiento es uniforme a lo largo de la curva, con celeridad constante $v 0$ . En el instante inicial la partícula se encuentra en $θ = 0$

Determine la ley horaria $θ = θ(t)$ .
Calcule el tiempo que tarda en llegar a 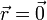$\vec{r}=\vec{0}$ . ¿Cuántas vueltas da para ello?
Halle el vector aceleración y sus componentes intrínsecas en cada punto de la trayectoria.
Determine la posición de los centros de curvatura de este movimiento.

2 Ley horaria

Para hallar la ley horaria $θ = θ(t)$ aplicamos que el movimiento es uniforme y por tanto

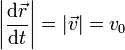
$\left|\frac{\mathrm{d}\vec{r}}{\mathrm{d}t}\right| = |\vec{v}| = v_0$

Sin embargo, lo que se nos da es la trayectoria como función de la coordenada $θ$ y la velocidad no es la derivada de la posición respecto a $θ$ , sino respecto al tiempo. Para relacionar las dos cosas aplicamos la regla de la cadena

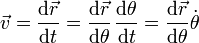
$\vec{v}=\frac{\mathrm{d}\vec{r}}{\mathrm{d}t}=\frac{\mathrm{d}\vec{r}}{\mathrm{d}\theta}\,\frac{\mathrm{d}\theta}{\mathrm{d}t}=\frac{\mathrm{d}\vec{r}}{\mathrm{d}\theta}\dot{\theta}$

Aquí 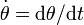$\dot{\theta} =\mathrm{d}\theta/\mathrm{d}t$ es una función que debemos determinar.

Tomando módulos

$v_0= \left|\vec{v}\right|= \left|\frac{\mathrm{d}\vec{r}}{\mathrm{d}\theta}\right|\dot{\theta}$

Derivando en la ecuación de la trayectoria

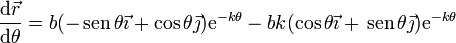
$\displaystyle\frac{\mathrm{d}\vec{r}}{\mathrm{d}\theta} = b(-\,\mathrm{sen}\,\theta\vec{\imath}+\cos\theta\vec{\jmath})\mathrm{e}^{-k\theta}-bk(\cos\theta\vec{\imath}+\,\mathrm{sen}\,\theta\vec{\jmath})\mathrm{e}^{-k\theta}$

Podemos simplificar esta expresión definiendo dos vectores unitarios

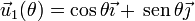
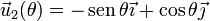
$\vec{u}_1(\theta) = \cos\theta\vec{\imath}+\,\mathrm{sen}\,\theta\vec{\jmath}$ $\vec{u}_2(\theta) = -\,\mathrm{sen}\,\theta\vec{\imath}+\cos\theta\vec{\jmath}$

Estos vectores verifican que son unitarios y ortogonales

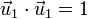
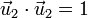
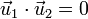
$\vec{u}_1\cdot\vec{u}_1=1$ $\vec{u}_2\cdot\vec{u}_2=1$ $\vec{u}_1\cdot\vec{u}_2=0$

y tienen por derivadas

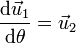
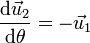
$\frac{\mathrm{d}\vec{u}_1}{\mathrm{d}\theta}=\vec{u}_2$ $\frac{\mathrm{d}\vec{u}_2}{\mathrm{d}\theta}=-\vec{u}_1$

Con ayuda de estos vectores, la posición se expresa

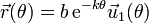
$\vec{r}(\theta)=b\,\mathrm{e}^{-k\theta}\vec{u}_1(\theta)$

y la derivada respecto al ángulo $θ$

$\frac{\mathrm{d}\vec{r}}{\mathrm{d}\theta}=-k b\,\mathrm{e}^{-k\theta}\vec{u}_1+b\,\mathrm{e}^{-k\theta}\frac{\mathrm{d}\vec{u}_1}{\mathrm{d}\theta}=b\,\mathrm{e}^{-k\theta}\left(\vec{u}_2-k\vec{u}_1\right)$

que es la expresión que ya teníamos, pero más concisa.

Imponiendo ahora que la celeridad es $v 0$ queda

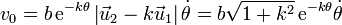
$v_0 = b\,\mathrm{e}^{-k\theta}\left|\vec{u}_2-k\vec{u}_1\right|\dot{\theta}=b\sqrt{1+k^2}\,\mathrm{e}^{-k\theta}\dot{\theta}$

Para integrar esta ecuación separamos los diferenciales

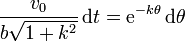
$\frac{v_0}{b\sqrt{1+k^2}}\,\mathrm{d}t = \mathrm{e}^{-k\theta}\,\mathrm{d}\theta$

e integramos, teniendo en cuenta que para $t = 0$ , $θ = 0$

$\frac{v_0}{b\sqrt{1+k^2}}\int_0^t\mathrm{d}t = \int_0^\theta \mathrm{e}^{-k\theta}\,\mathrm{d}\theta$

lo que nos da

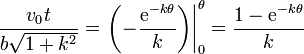
$\frac{v_0 t}{b\sqrt{1+k^2}} = \left.\left(-\frac{\mathrm{e}^{-k\theta}}{k}\right)\right|_0^\theta = \frac{1-\mathrm{e}^{-k\theta}}{k}$

Despejando de aquí

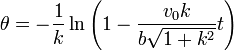
$\theta = -\frac{1}{k}\ln\left(1-\frac{v_0k}{b\sqrt{1+k^2}}t\right)$

3 Tiempo en llegar al origen

El tiempo que tarda la partícula en llegar al origen lo obtenemos exigiendo que 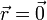$\vec{r}=\vec{0}$ . La posición para cada valor de $θ$ es igual a

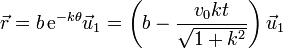
$\vec{r}=b\,\mathrm{e}^{-k\theta}\vec{u}_1 = \left(b-\frac{v_0 k t}{\sqrt{1+k^2}}\right)\vec{u}_1$

Este vector se anulará en

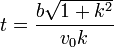
$t = \frac{b\sqrt{1+k^2}}{v_0k}$

Este es el tiempo en llegar al centro.

Nótese que este valor de $t$ corresponde a un valor de 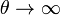$\theta\to\infty$ . Puesto que $θ$ representa el ángulo que el vector de posición forma con el eje X y que una circunferencia completa significa aumentar $θ$ en $2π$ , vemos que la partícula da infinitas vueltas antes de llegar al origen, aunque para ello requiere un tiempo finito. Igualmente, aunque de infinitas vueltas, la distancia recorrida por la partícula es finita e igual a

$\Delta s = v_0 t = \frac{b\sqrt{1+k^2}}{k}$

Esto es posible porque las suscesivas vueltas son cada vez más pequeñas.

4 Aceleración

Para el cálculo de la aceleración tenemos un problema similar al de la velocidad. La cuestión es que la aceleracion no es la derivada de la velocidad respecto a $θ$ , sino respecto al tiempo, con lo que hay que tener mucho cuidado con qué seriva y respecto a qué.

Partimos de las expresiones que ya conocemos para la velocidad

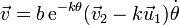
$\vec{v}=b\,\mathrm{e}^{-k\theta}(\vec{v}_2-k\vec{u}_1)\dot{\theta}$

Conocemos también la ley horaria

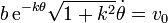
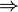
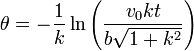
$b\,\mathrm{e}^{-k\theta}\sqrt{1+k^2}\dot{\theta}=v_0$ $\Rightarrow$ $\theta=-\frac{1}{k}\ln\left(\frac{v_0kt}{b\sqrt{1+k^2}}\right)$

Una posibilidad sería entonces sustituir $θ(t)$ en la expresión de la velocidad y derivar lo que salga respecto al tiempo. Sin embargo debido a que en esta fórmula aparecen seos y cosenos de logaritmos, la posibilidad de equivocarse al derivar es muy alta.

La solución consiste en aplicar de nuevo la regla de la cadena. Vamos a considerar la velocidad como función del ángulo $θ$ y calcular la aceleración como

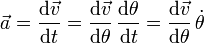
$\vec{a}=\frac{\mathrm{d}\vec{v}}{\mathrm{d}t}=\frac{\mathrm{d}\vec{v}}{\mathrm{d}\theta}\,\frac{\mathrm{d}\theta}{\mathrm{d}t}=\frac{\mathrm{d}\vec{v}}{\mathrm{d}\theta}\,\dot{\theta}$

Para ello necesitamos, en primer lugar la velocidad como función de $θ$ , que es algo que aun no tenemos. En la expresión de arriba aparece la velocidad como función de $θ$ y de $\dot{\theta}$

5 Centros de curvatura

@@ Línea 100: / Línea 100: @@
 <center><math>b\,\mathrm{e}^{-k\theta}\sqrt{1+k^2}\dot{\theta}=v_0</math>{{tose}}<math>\theta=-\frac{1}{k}\ln\left(\frac{v_0kt}{b\sqrt{1+k^2}}\right)</math></center>
+Una posibilidad sería entonces sustituir <math>\theta(t)</math> en la expresión de la velocidad y derivar lo que salga respecto al tiempo. Sin embargo debido a que en esta fórmula aparecen seos y cosenos de logaritmos, la posibilidad de equivocarse al derivar es muy alta.
+La solución consiste en aplicar de nuevo la regla de la cadena. Vamos a considerar la velocidad como función del ángulo <math>\theta</math> y calcular la aceleración como
+<center><math>\vec{a}=\frac{\mathrm{d}\vec{v}}{\mathrm{d}t}=\frac{\mathrm{d}\vec{v}}{\mathrm{d}\theta}\,\frac{\mathrm{d}\theta}{\mathrm{d}t}=\frac{\mathrm{d}\vec{v}}{\mathrm{d}\theta}\,\dot{\theta}</math></center>
+Para ello necesitamos, en primer lugar la velocidad como función de <math>\theta</math>, que es algo que aun no tenemos. En la expresión de arriba aparece la velocidad como función de <math>\theta</math> y de <math>\dot{\theta}</math>
 ==Centros de curvatura==
 [[Categoría:Problemas de cinemática de la partícula]]

Espiral logarítmica

De Laplace

Revisión de 16:08 25 jun 2010

Contenido

1 Enunciado

2 Ley horaria

3 Tiempo en llegar al origen

4 Aceleración

5 Centros de curvatura

Herramientas:

Buscar

Vistas

Herramientas

Herramientas personales

Navegación

SEARCH

TOOLBOX

LANGUAGES