4.6. Identificación de posibles movimientos rígidos

De Laplace

(Redirigido desde 5.6. Identificación de posibles movimientos rígidos)

Contenido

1 Enunciado
2 Introducción
3 Caso I
4 Caso II
5 Caso III
6 Caso IV
7 Caso V
8 Caso VI
9 Resumen

1 Enunciado

En un hipotético sólido rígido, consideramos los puntos

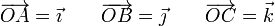
$\overrightarrow{OA}=\vec{\imath}\qquad\overrightarrow{OB}=\vec{\jmath}\qquad\overrightarrow{OC}=\vec{k}$

y analizamos los casos correspondientes a las siguientes velocidades para los tres puntos:

Caso	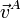$\vec{v}^A$	$\vec{v}^B$	$\vec{v}^C$
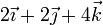
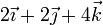
I	$2\vec{\imath}+2\vec{\jmath}+4\vec{k}$	$2\vec{\imath}+2\vec{\jmath}+4\vec{k}$	$2\vec{k}$
II	$2\vec{\imath}+\vec{\jmath}+2\vec{k}$	$3\vec{\imath}+2\vec{\jmath}$	$2\vec{\imath}+4\vec{\jmath} + 2\vec{k}$
III	$2\vec{\imath}+2\vec{\jmath}+2\vec{k}$	$2\vec{\imath}+2\vec{\jmath}+2\vec{k}$	$2\vec{\imath}+2\vec{\jmath}+2\vec{k}$
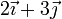
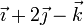
IV	$2\vec{\imath}+3\vec{\jmath}$	$\vec{\imath}+2\vec{\jmath}-\vec{k}$	$4\vec{\imath}+4\vec{\jmath}+2\vec{k}$
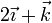
V	$2\vec{\imath}+\vec{k}$	$4\vec{\imath}+2\vec{\jmath}+3\vec{k}$	$3\vec{\imath}+\vec{\jmath}+2\vec{k}$
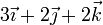
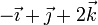
VI	$2\vec{\imath}+\vec{\jmath}+2\vec{k}$	$3\vec{\imath}+2\vec{\jmath}+2\vec{k}$	$-\vec{\imath}+\vec{\jmath}+2\vec{k}$

Todas las cantidades están expresadas en las unidades del SI.

Identifique cuáles de las situaciones anteriores son compatibles con la condición de rigidez. Para las que sí lo son, identifique si se trata de un movimiento de traslación pura, rotación pura o helicoidal.

2 Introducción

Para identificar los diferentes estados de movimiento, conocidas las velocidades de tres puntos no alineados, podemos seguir el siguiente esquema:

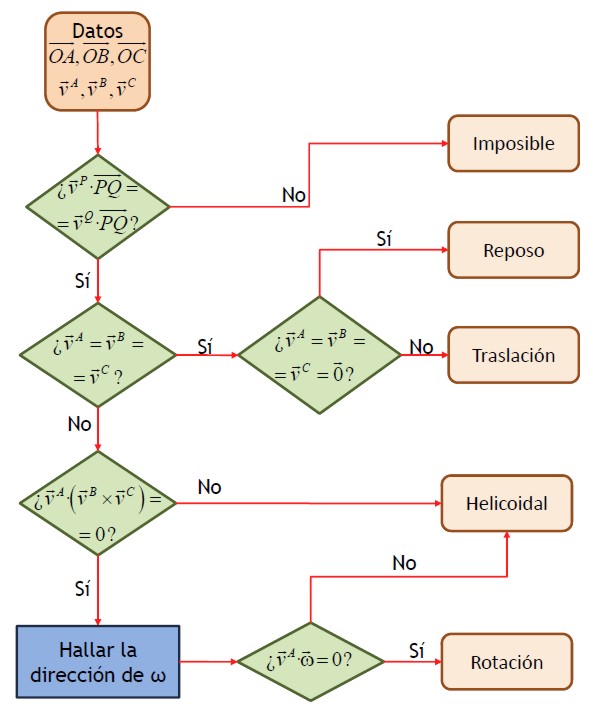
3 Caso I

Tenemos las velocidades

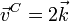
$\vec{v}^A=2\vec{\imath}+2\vec{\jmath}+4\vec{k}$ $\vec{v}^B=2\vec{\imath}+2\vec{\jmath}+4\vec{k}$ $\vec{v}^C=2\vec{k}$

Comprobamos primero si se trata de un posible movimiento rígido, chequeando la condición de equiproyectividad para cada par de puntos

Partículas A y B

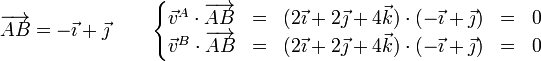
$\overrightarrow{AB}=-\vec{\imath}+\vec{\jmath}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AB} & = & (2\vec{\imath}+2\vec{\jmath}+4\vec{k})\cdot(-\vec{\imath}+\vec{\jmath}) & = & 0 \\ \vec{v}^B\cdot\overrightarrow{AB} & = & (2\vec{\imath}+2\vec{\jmath}+4\vec{k})\cdot(-\vec{\imath}+\vec{\jmath}) & = & 0 \end{matrix}\right.$

Partículas A y C

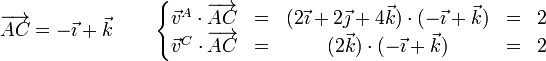
$\overrightarrow{AC}=-\vec{\imath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AC} & = & (2\vec{\imath}+2\vec{\jmath}+4\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & 2 \\ \vec{v}^C\cdot\overrightarrow{AC} & = & (2\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & 2 \end{matrix}\right.$

Partículas B y C

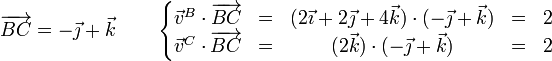
$\overrightarrow{BC}=-\vec{\jmath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^B\cdot\overrightarrow{BC} & = & (2\vec{\imath}+2\vec{\jmath}+4\vec{k})\cdot(-\vec{\jmath}+\vec{k}) & = & 2 \\ \vec{v}^C\cdot\overrightarrow{BC} & = & (2\vec{k})\cdot(-\vec{\jmath}+\vec{k}) & = & 2 \end{matrix}\right.$

Se verifica en los tres casos, por lo que se trata de un movimiento posible para un sólido rígido.

A continuación, comprobamos si las tres velocidades son iguales. No lo son. Por tanto, no puede tratarse de una traslación o un estado de reposo.

Vemos ahora si las velocidades son coplanarias

$\vec{v}^A\cdot(\vec{v}^B\times\vec{v}^C)=\left|\begin{matrix}2 & 2 & 4 \\ 2 & 2 & 4 \\ 0 & 0 & 2\end{matrix}\right|=0$

Al haber dos velocidades iguales, necesariamente los tres vectores son coplanarios. Necesitamos hallar entonces la dirección de la velocidad angular. Esto es fácil; al haber dos velocidades iguales, la velocidad angular va en la dirección de la recta que pasa por esos dos puntos. En este caso

$\vec{\omega} = \lambda\overrightarrow{AB}=\lambda(-\vec{\imath}+\vec{\jmath})$

y comprobamos ahora si alguna de las velocidades es perpendicular a esta dirección. No necesitamos volver a calcular nada, ya que lo hicimos previamente:

$\vec{v}^A\cdot\overrightarrow{AB}=0$

Por tanto se trata de un movimiento de rotación pura.

4 Caso II

Tenemos las velocidades

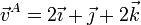
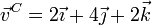
$\vec{v}^A=2\vec{\imath}+\vec{\jmath}+2\vec{k}$ $\vec{v}^B=3\vec{\imath}+2\vec{\jmath}$ $\vec{v}^C=2\vec{\imath}+4\vec{\jmath} + 2\vec{k}$

Comprobamos la equiproyectividad

Partículas A y B

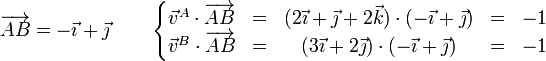
$\overrightarrow{AB}=-\vec{\imath}+\vec{\jmath}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AB} & = & (2\vec{\imath}+\vec{\jmath}+2\vec{k})\cdot(-\vec{\imath}+\vec{\jmath}) & = & -1 \\ \vec{v}^B\cdot\overrightarrow{AB} & = & (3\vec{\imath}+2\vec{\jmath})\cdot(-\vec{\imath}+\vec{\jmath}) & = & -1 \end{matrix}\right.$

Partículas A y C

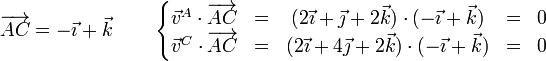
$\overrightarrow{AC}=-\vec{\imath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AC} & = & (2\vec{\imath}+\vec{\jmath}+2\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & 0 \\ \vec{v}^C\cdot\overrightarrow{AC} & = & (2\vec{\imath}+4\vec{\jmath} + 2\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & 0 \end{matrix}\right.$

Partículas B y C

$\overrightarrow{BC}=-\vec{\jmath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^B\cdot\overrightarrow{BC} & = & (3\vec{\imath}+2\vec{\jmath})\cdot(-\vec{\jmath}+\vec{k}) & = & -2 \\ \vec{v}^C\cdot\overrightarrow{BC} & = & (2\vec{\imath}+4\vec{\jmath} + 2\vec{k})\cdot(-\vec{\jmath}+\vec{k}) & = & -2 \end{matrix}\right.$

De nuevo se verifica en los tres casos, por lo que se trata de un movimiento posible.

Puesto que las tres velocidades no son iguales, no puede tratarse de una traslación o un estado de reposo.

Verificamos entonces si las velocidades son coplanarias:

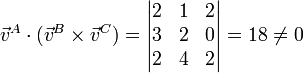
$\vec{v}^A\cdot(\vec{v}^B\times\vec{v}^C) = \left|\begin{matrix} 2 & 1 & 2 \\ 3 & 2 & 0 \\ 2 & 4 & 2 \end{matrix}\right| = 18\neq 0$

Por no ser nulo, se trata de un movimiento helicoidal.

5 Caso III

El tercer caso

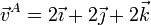
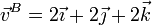
$\vec{v}^A=2\vec{\imath}+2\vec{\jmath}+2\vec{k}$ $\vec{v}^B=2\vec{\imath}+2\vec{\jmath}+2\vec{k}$ $\vec{v}^C=2\vec{\imath}+2\vec{\jmath} + 2\vec{k}$

puede clasificarse por simple inspección. Puesto que las tres velocidades son iguales, y no nulas, se trata de un movimiento de traslación.

6 Caso IV

En este caso, las velocidades valen

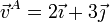
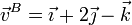
$\vec{v}^A=2\vec{\imath}+3\vec{\jmath}$ $\vec{v}^B=\vec{\imath}+2\vec{\jmath}-\vec{k}$ $\vec{v}^C=4\vec{\imath}+4\vec{\jmath}+2\vec{k}$

Examinamos la condición de rigidez

Partículas A y B

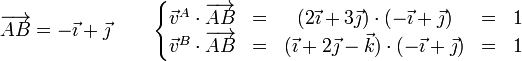
$\overrightarrow{AB}=-\vec{\imath}+\vec{\jmath}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AB} & = & (2\vec{\imath}+3\vec{\jmath})\cdot(-\vec{\imath}+\vec{\jmath}) & = & 1 \\ \vec{v}^B\cdot\overrightarrow{AB} & = & (\vec{\imath}+2\vec{\jmath}-\vec{k})\cdot(-\vec{\imath}+\vec{\jmath}) & = & 1 \end{matrix}\right.$

Partículas A y C

$\overrightarrow{AC}=-\vec{\imath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AC} & = & (2\vec{\imath}+3\vec{\jmath})\cdot(-\vec{\imath}+\vec{k}) & = & -2 \\ \vec{v}^C\cdot\overrightarrow{AC} & = & (4\vec{\imath}+4\vec{\jmath}+2\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & -2 \end{matrix}\right.$

Partículas B y C

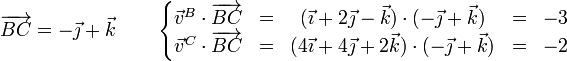
$\overrightarrow{BC}=-\vec{\jmath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^B\cdot\overrightarrow{BC} & = & (\vec{\imath}+2\vec{\jmath}-\vec{k})\cdot(-\vec{\jmath}+\vec{k}) & = & -3 \\ \vec{v}^C\cdot\overrightarrow{BC} & = & (4\vec{\imath}+4\vec{\jmath}+2\vec{k})\cdot(-\vec{\jmath}+\vec{k}) & = & -2 \end{matrix}\right.$

Puesto que no se cumple la condición de equiproyectividad, este caso es imposible como movimiento de un sólido rígido.

7 Caso V

En este caso, tenemos las velocidades

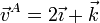
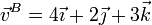
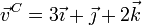
$\vec{v}^A=2\vec{\imath}+\vec{k}$ $\vec{v}^B=4\vec{\imath}+2\vec{\jmath}+3\vec{k}$ $\vec{v}^C=3\vec{\imath}+\vec{\jmath}+2\vec{k}$

Examinamos la equiproyectividad

Partículas A y B

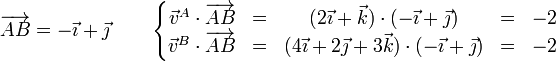
$\overrightarrow{AB}=-\vec{\imath}+\vec{\jmath}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AB} & = & (2\vec{\imath}+\vec{k})\cdot(-\vec{\imath}+\vec{\jmath}) & = & -2 \\ \vec{v}^B\cdot\overrightarrow{AB} & = & (4\vec{\imath}+2\vec{\jmath}+3\vec{k})\cdot(-\vec{\imath}+\vec{\jmath}) & = & -2 \end{matrix}\right.$

Partículas A y C

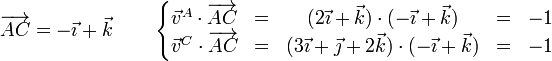
$\overrightarrow{AC}=-\vec{\imath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AC} & = & (2\vec{\imath}+\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & -1\\ \vec{v}^C\cdot\overrightarrow{AC} & = & (3\vec{\imath}+\vec{\jmath}+2\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & -1 \end{matrix}\right.$

Partículas B y C

$\overrightarrow{BC}=-\vec{\jmath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^B\cdot\overrightarrow{BC} & = & (4\vec{\imath}+2\vec{\jmath}+3\vec{k})\cdot(-\vec{\jmath}+\vec{k}) & = & 1 \\ \vec{v}^C\cdot\overrightarrow{BC} & = & (3\vec{\imath}+\vec{\jmath}+2\vec{k})\cdot(-\vec{\jmath}+\vec{k}) & = & 1 \end{matrix}\right.$

De nuevo se verifica en los tres casos, por lo que se trata de un movimiento posible.

Las tres velocidades no son iguales, por lo que no puede tratarse de una traslación o un estado de reposo.

Verificamos entonces si las velocidades son coplanarias:

$\vec{v}^A\cdot(\vec{v}^B\times\vec{v}^C) = \left|\begin{matrix} 2 & 0 & 1 \\ 4 & 2 & 3 \\ 3 & 1 & 2 \end{matrix}\right| = 0$

Puesto que es nulo, debemos ahora determinar la dirección de la velocidad angular. Para ello suponemos una velocidad angular de la forma

$\vec{\omega} = \omega_x\vec{\imath}+\omega_y\vec{\jmath}+\omega_\vec{k}$

y sustituimos en las relaciones

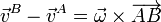
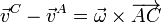
$\vec{v}^B-\vec{v}^A = \vec{\omega}\times\overrightarrow{AB}$ $\vec{v}^C-\vec{v}^A = \vec{\omega}\times\overrightarrow{AC}$

Para el par A y B queda

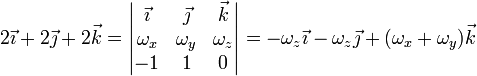
$2\vec{\imath}+2\vec{\jmath}+2\vec{k}=\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ \omega_x & \omega_y & \omega_z\\ -1 & 1 & 0 \end{matrix}\right| = -\omega_z\vec{\imath} -\omega_z \vec{\jmath}+(\omega_x +\omega_y)\vec{k}$

de donde

$\omega_x+\omega_y = 2\qquad\qquad\omega_z = -2$

Repitiendo para A y C

$\vec{\imath}+\vec{\jmath}+\vec{k}=\left|\begin{matrix}\vec{\imath} & \vec{\jmath} & \vec{k} \\ \omega_x & \omega_y & \omega_z\\ -1 & 0 & 1 \end{matrix}\right| = \omega_y\vec{\imath} -(\omega_x+\omega_z) \vec{\jmath}+\omega_y\vec{k}$

de donde

$\omega_x+\omega_z = -1\qquad\qquad\omega_y = 1$

lo que nos da la velocidad angular

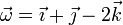
$\vec{\omega}=\vec{\imath}+\vec{\jmath}-2\vec{k}$

Comprobamos ahora si cualquiera de las velocidades es perpendicular a esta velocidad angular

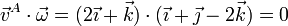
$\vec{v}^A\cdot\vec{\omega} = (2\vec{\imath}+\vec{k})\cdot(\vec{\imath}+\vec{\jmath}-2\vec{k})= 0$

Puesto que la velocidad lineal es perpendicular a la velocidad angular, la velocidad de deslizamiento es nula y el movimiento es de rotación pura.

8 Caso VI

Por último, tenemos las velocidades,

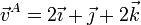
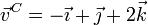
$\vec{v}^A=2\vec{\imath}+\vec{\jmath}+2\vec{k}$ $\vec{v}^B=3\vec{\imath}+2\vec{\jmath}+2\vec{k}$ $\vec{v}^C=-\vec{\imath}+\vec{\jmath}+2\vec{k}$

Testeamos la condición de rigidez

Partículas A y B

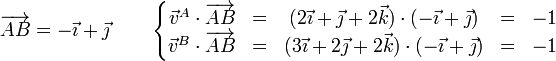
$\overrightarrow{AB}=-\vec{\imath}+\vec{\jmath}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AB} & = & (2\vec{\imath}+\vec{\jmath}+2\vec{k})\cdot(-\vec{\imath}+\vec{\jmath}) & = & -1 \\ \vec{v}^B\cdot\overrightarrow{AB} & = & (3\vec{\imath}+2\vec{\jmath}+2\vec{k})\cdot(-\vec{\imath}+\vec{\jmath}) & = & -1 \end{matrix}\right.$

Partículas A y C

$\overrightarrow{AC}=-\vec{\imath}+\vec{k}\qquad\left\{\begin{matrix}\vec{v}^A\cdot\overrightarrow{AC} & = & (2\vec{\imath}+\vec{\jmath}+2\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & 0 \\ \vec{v}^C\cdot\overrightarrow{AC} & = & (-\vec{\imath}+\vec{\jmath}+2\vec{k})\cdot(-\vec{\imath}+\vec{k}) & = & 3 \end{matrix}\right.$

No necesitamos continuar. Una vez que se viola la equiproyectividad podemos afirmar que se trata de un movimiento imposible para un sólido rígido.

9 Resumen

Reuniendo los resultados de los seis casos queda

Caso	Estado
I	Rotación
II	Helicoidal
III	Traslación
IV	Imposible
V	Rotación
VI	Imposible

4.6. Identificación de posibles movimientos rígidos

De Laplace

Contenido

1 Enunciado

2 Introducción

3 Caso I

4 Caso II

5 Caso III

6 Caso IV

7 Caso V

8 Caso VI

9 Resumen

Herramientas:

Buscar

Vistas

Herramientas

Herramientas personales

Navegación

SEARCH

TOOLBOX

LANGUAGES